For an operator (A=a_{mu} X_{mu}) corresponding to a linear combination of generators (X_{mu}) for a Lie algebra,
Question:
For an operator \(A=a_{\mu} X_{\mu}\) corresponding to a linear combination of generators \(X_{\mu}\) for a Lie algebra, use Eq. (7.10) and the Jacobi identity (3.6) to prove that
\[\left[A,\left[E_{\alpha}, E_{\beta}\right]\right]=(\alpha+\beta)\left[E_{\alpha}, E_{\beta}\right],\]
where \(E_{\alpha}\) and \(E_{\beta}\) are generators that are not in the Cartan subalgebra.
Data from Eq. 7.10
![]()
Data from Eq. 3.6
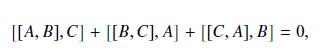
Fantastic news! We've Found the answer you've been seeking!
Step by Step Answer:
Related Book For 

Symmetry Broken Symmetry And Topology In Modern Physics A First Course
ISBN: 9781316518618
1st Edition
Authors: Mike Guidry, Yang Sun
Question Posted:





