For orbital angular momentum $L$, spin angular momentum $S$, total angular momentum $J$, and projection $M$ of
Question:
For orbital angular momentum $L$, spin angular momentum $S$, total angular momentum $J$, and projection $M$ of the total angular momentum, use the Wigner-Eckart theorem to express $\left\langle L S J M\left|L_{z}+2 S_{z}\right| L S J M\rightangle$ in terms of reduced matrix elements. Evaluate the reduced matrix elements to show that $\left\langle L S J M\left|L_{z}+2 S_{z}\right| L S J M\rightangle=M g$, where the Landé $g$-factor is
\[g \equiv 1+\frac{J(J+1)+S(S+1)-L(L+1)}{2 J(J+1)} .\]
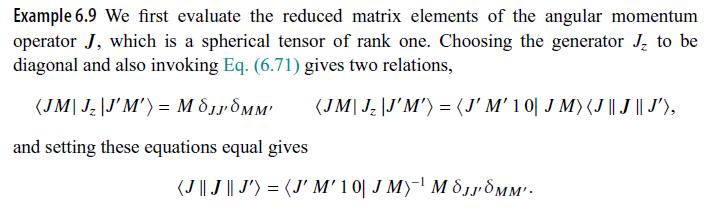
Consult Example 6.9, and note that $L$ operates only on the orbital part and $S$ only on the spin part of the wavefunction, so Eq. (30.12) is appropriate.
Data from Example 6.9
Data from Eq. 30.12
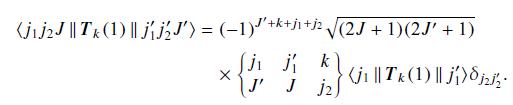
Step by Step Answer:

Symmetry Broken Symmetry And Topology In Modern Physics A First Course
ISBN: 9781316518618
1st Edition
Authors: Mike Guidry, Yang Sun





