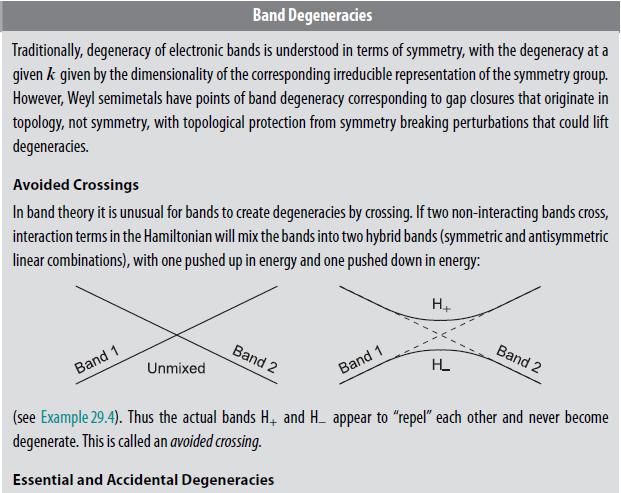
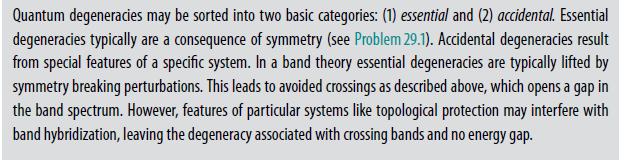
The normal (not accidental; see Box 29.2) degeneracies in a quantum system result from symmetry. Show that if a Hamiltonian $H$ is invariant under transformation by a unitary symmetry operator $S$, then (a) $H$ commutes with $S$, and (b) if $|\alphaangle$ is an eigenstate of $H$ with energy $E$, then the symmetry transformed state $|\betaangle=S|\alphaangle$ is also an eigenstate with energy $E$. Thus, if $|\alphaangle$ and $|\betaangle$ are linearly independent they define states that are degenerate in energy because they are related by symmetry.

Data from Box 29.2

Transcribed Image Text:

Band Degeneracies Traditionally, degeneracy of electronic bands is understood in terms of symmetry, with the degeneracy at a given k given by the dimensionality of the corresponding irreducible representation of the symmetry group. However, Weyl semimetals have points of band degeneracy corresponding to gap closures that originate in topology, not symmetry, with topological protection from symmetry breaking perturbations that could lift degeneracies. Avoided Crossings In band theory it is unusual for bands to create degeneracies by crossing. If two non-interacting bands cross, interaction terms in the Hamiltonian will mix the bands into two hybrid bands (symmetric and antisymmetric linear combinations), with one pushed up in energy and one pushed down in energy: Band 1 Unmixed Band 2 Band 1 H+ Band 2 H_ (see Example 29.4). Thus the actual bands H+ and H_ appear to "repel" each other and never become degenerate. This is called an avoided crossing. Essential and Accidental Degeneracies