Apply the conditions of steady state and solenoidal vector fields (mathbf{y}) to the Hopf fde for cylindrical
Question:
Apply the conditions of steady state and solenoidal vector fields \(\mathbf{y}\) to the Hopf fde for cylindrical coordinates in the flow domain \(\mathcal{D}\) obtained in the previous Problem (10.2).
10.3.1 Specialize the result to constant \(\mathbf{G}\) and \(\frac{\partial P_{0}}{\partial z}\).
10.3.2 Show that the scalar product of two modes in \(\mathcal{B}_{e}\) is reduced to an integral w.r.t. the radial coordinate.
Problem (10.2)
Transform the Hopf fde (9.40) to cylindrical coordinates in \(\mathcal{D}\). The explicit form of the pressure gradient term as functional of the velocity is not required.
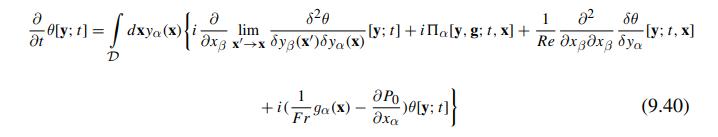
Fantastic news! We've Found the answer you've been seeking!
Step by Step Answer:
Related Book For 

Navier Stokes Turbulence Theory And Analysis
ISBN: 9783030318697
1st Edition
Authors: Wolfgang Kollmann
Question Posted:





