Consider the flow through a straight, circular pipe periodic in axial direction. A solenoidal ONS basis \(\mathcal{B}_{e}\) was constructed in Sect. 25.21 spanning the test function space \(\mathcal{N}\) for the characteristic functional \(\theta[\mathbf{y} ; t], \mathbf{y} \in \mathcal{N}\). Solve the BVP for the projected characteristic functional \(\theta_{N}\left(y_{0,0,-\frac{1}{2} N}, \ldots, y_{\frac{1}{2} N, N, \frac{1}{2} N}\right)\) introduced in Sect. 10.4 w.r.t. the basis \(\mathcal{B}_{e}\).

10.4.1 Apply the pde (10.21) to the single mode \(N=0\), set up the boundary condition and solve it for \(y \geq 0\).

10.4.2 Compute the coefficients \(a_{k, n, m}^{l, o, q, r, s, t}\) in \(A_{k, n, m}^{r, s, t}=\sum_{l, o, q} y_{l, o, q} a_{k, n, m}^{l, o, q, r, s, t}\) and \(C_{z}^{0, n, 0}\) in \(C\left(y_{0, n, 0}\right)=\frac{\partial P_{0}}{\partial z} \sum_{n=0}^{N} C_{z}^{0, n, 0} y_{0, n, 0}\) the pde (10.21) for several modes, say \(N=2\) and plot the results.

Sect. 10.4

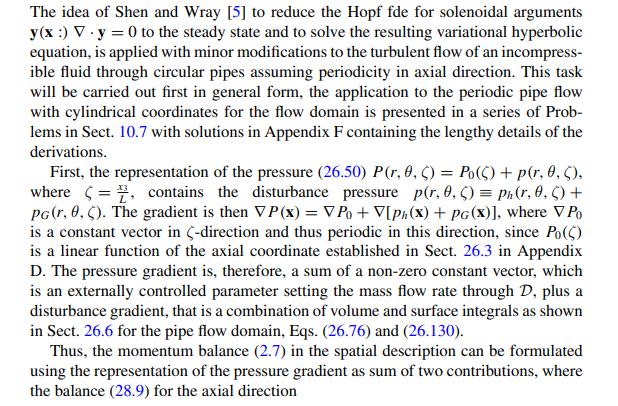
pde (10.21)

Transcribed Image Text:

The idea of Shen and Wray [5] to reduce the Hopf fde for solenoidal arguments y(x) V y = 0 to the steady state and to solve the resulting variational hyperbolic equation, is applied with minor modifications to the turbulent flow of an incompress- ible fluid through circular pipes assuming periodicity in axial direction. This task will be carried out first in general form, the application to the periodic pipe flow with cylindrical coordinates for the flow domain is presented in a series of Prob- lems in Sect. 10.7 with solutions in Appendix F containing the lengthy details of the derivations. First, the representation of the pressure (26.50) P(r, 0, 0) = Po(C) + P(r, 0, C), where contains the disturbance pressure p(r. 0, () = ph(r, 0, () + PG(r, 0, C). The gradient is then VP(x) = V Po+ V[ph(x) + PG(x)], where V Po is a constant vector in (-direction and thus periodic in this direction, since Po(C) is a linear function of the axial coordinate established in Sect. 26.3 in Appendix D. The pressure gradient is, therefore, a sum of a non-zero constant vector, which is an externally controlled parameter setting the mass flow rate through D, plus a disturbance gradient, that is a combination of volume and surface integrals as shown in Sect. 26.6 for the pipe flow domain, Eqs. (26.76) and (26.130). Thus, the momentum balance (2.7) in the spatial description can be formulated using the representation of the pressure gradient as sum of two contributions, where the balance (28.9) for the axial direction