Compute the Gateaux derivative with respect to \(v_{\alpha}(\mathbf{x}, t)\) of the pressure represented in terms of the Green's function as shown in Sect. 11.1 for nonhomogeneous Neumann conditions for the pressure and homogeneous Dirichlet boundary conditions for the momentum balances. Specialize the Gateaux derivative to harmonic directions.

Sect. 11.1

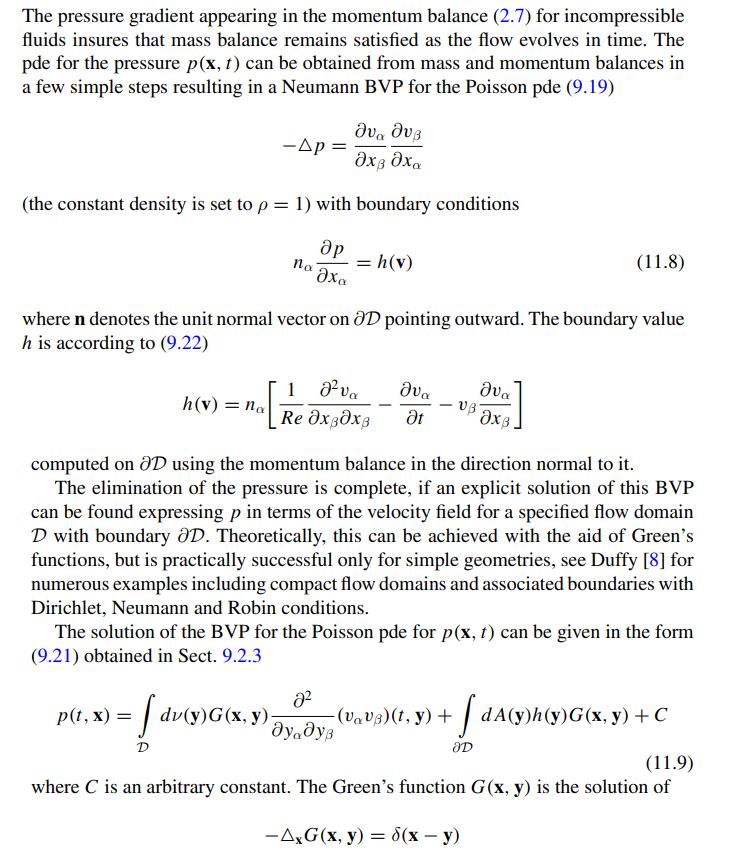
Transcribed Image Text:

The pressure gradient appearing in the momentum balance (2.7) for incompressible fluids insures that mass balance remains satisfied as the flow evolves in time. The pde for the pressure p(x, t) can be obtained from mass and momentum balances in a few simple steps resulting in a Neumann BVP for the Poisson pde (9.19) -Ap= (the constant density is set to p = 1) with boundary conditions na = =h(v) (11.8) where n denotes the unit normal vector on D pointing outward. The boundary value h is according to (9.22) 1 Jva ava - - h(v) (v) = na Re Ox30x3 t computed on D using the momentum balance in the direction normal to it. The elimination of the pressure is complete, if an explicit solution of this BVP can be found expressing p in terms of the velocity field for a specified flow domain D with boundary D. Theoretically, this can be achieved with the aid of Green's functions, but is practically successful only for simple geometries, see Duffy [8] for numerous examples including compact flow domains and associated boundaries with Dirichlet, Neumann and Robin conditions. The solution of the BVP for the Poisson pde for p(x, t) can be given in the form (9.21) obtained in Sect. 9.2.3 p(t, x) = = dv (y)G 82 dv(y)G(x, y)- (Vavg)(t, y) + dA(y)h(y)G(x, y) +C S JD (11.9) where C is an arbitrary constant. The Green's function G(x, y) is the solution of -AxG(x, y) = (x y)