Consider the following system of ordinary differential equations

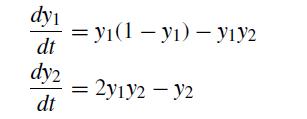
with y₁(0) = 0.5 and y₂(0) = 5. We consider the explicit Euler method for numerical integration. What is the maximum value for the time step h that guarantees numerically stable integration over a single time step? Do you expect the integration to be stiff in the vicinity of t = 0? Explain.

Transcribed Image Text:

dy1 dt dy2 = = 2y1y2 - y2 dt = yı(1-yi)- y1y2