1. Let : R m R k and g : R n R m...
Question:
1. Let ƒ : Rm → Rk and g : Rn → Rm be two maps. Let h : Rn→ Rk be the composite map h = ƒ ο g, with values h(x) = ƒ(g(x)) for x ∈ Rn. Show that the derivatives of h can be expressed via a matrix-matrix product, as Jh(x) = Jƒ (g(x)) · Jg(x), where Jh(x) is the Jacobian matrix of h at x, i.e., the matrix whose (i, j) element is 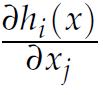 .
.
2. Let g be an affine map of the form g(x) = Ax + b, for A ∈ Rm,n, b ∈ Rm. Show that the Jacobian of h(x) = ƒ(g(x)) is
![]()
3. Let g be an affine map as in the previous point, let ƒ: Rn → R (a scalar-valued function), and let h(x) = ƒ(g(x)). Show that
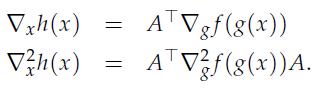
Fantastic news! We've Found the answer you've been seeking!
Step by Step Answer:
Related Book For 

Optimization Models
ISBN: 9781107050877
1st Edition
Authors: Giuseppe C. Calafiore, Laurent El Ghaoui
Question Posted:





