In this exercise, we revisit Exercise 9.3, and approach it using the S-procedure of Section 11.3.3.1. 1.
Question:
In this exercise, we revisit Exercise 9.3, and approach it using the S-procedure of Section 11.3.3.1.
1. Show that the minimum distance from the line segment L to the origin is above a given number R ≥ 0 if and only if
![]()
2. Apply the S-procedure, and prove that the above is in turn equivalent to the LMI in ![]()
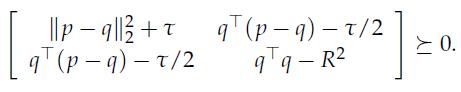
3. Using the Schur complement rule15, show that the above is consistent with the result given in Exercise 9.3.
Section 11.3.3.1:
The S-procedure. The so-called S-procedure establishes an equivalence between a certain LMI condition and an implication between two quadratic functions. More precisely, let f0(x), f1(x) be two quadratic functions:
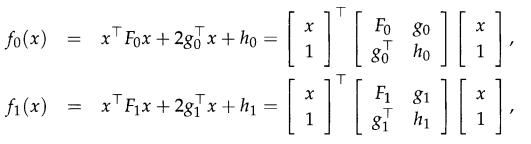
Fantastic news! We've Found the answer you've been seeking!
Step by Step Answer:
Related Book For 

Optimization Models
ISBN: 9781107050877
1st Edition
Authors: Giuseppe C. Calafiore, Laurent El Ghaoui
Question Posted:





