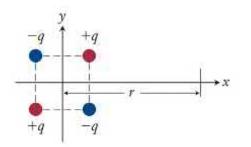
An electric quadrupole can be constructed by placing four charged objects at the corners of a square (Figure P23.49). The objects are identical except for the charge they carry: The two objects in one diagonally opposed pair each carry charge \(+q\), and the other two objects carry charge \(-q\). (Note that there is no charge on the quadrupole overall and that the combination of four charges has no dipole moment.) How does the magnitude of the electric field depend on the distance \(r\) from the center of the quadrupole? Take the side length for the square to be \(d\) and assume \(r \gg d\).

Data from Figure P23.49

+9 +9 -9 x