1. Dave is not absolutely sure that the "side" constraint of at least as much gas A as gas B is necessary. What is this constraint costing the company? That is, how much more revenue could Jansen earn if this constraint were ignored?

2. Dave consults the chemical experts, and they suggest that gas B could be produced with a "medium" level of TEL. The octane ratings for each feedstock with this medium level would be halfway between their low and high TEL octane ratings. Would this be a better option in terms of its optimal revenue?

3. Suppose that because of air pollution concerns, Jansen might have to lower the Reid vapor pressure maximum on each gas type (by the same amount). Use SolverTable to explore how such a change would affect Jansen's optimal revenue.

4. Dave believes the minimum required octane rating for gas A is too low. He would like to know how much this minimum rating could be increased before there would be no feasible solution (still assuming that gas A uses the low TEL level).

5. Dave suspects that only the relative prices matter in the optimal blending plan. Specifically, he believes that if all unit prices of the gas types and all unit values of the feedstocks increase by the same percentage, then the optimal blending plan will remain the same. Is he correct?

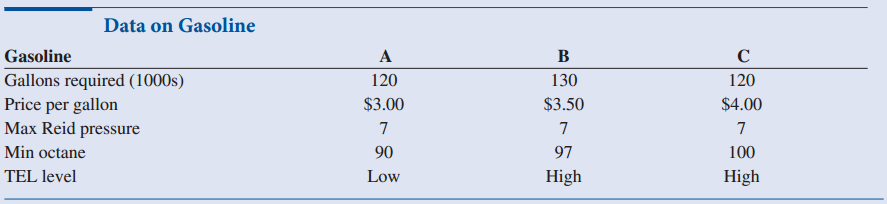
Jansen Gas creates three types of aviation gasoline (avgas), labeled A, B, and C. It does this by blending four feedstocks: Alkylate; Catalytic Cracked Gasoline; Straight Run Gasoline; and Isopentane. Jansen's production manager, Dave Wagner, has compiled the data on feedstocks and gas types in Tables 4.6 and 4.7. Table 4.6 lists the availabilities and values of the feedstocks, as well as their key chemical properties, Reid vapor pressure, and octane rating. Table 4.7 lists the gallons required, the prices, and chemical requirements of the three gas types.

Note that each feedstock can have either a low or a high level of TEL, which stands for tetraethyl lead. This is measured in units of milliliters per gallon, so that a low level might be 0.5 and a high level might be 4.0. (For this problem, the actual numbers do not matter.) As indicated in Table 4.6, the TEL level affects only the octane rating, not the Reid vapor pressure. Also, gas A is always made with a low TEL level, whereas gas types B and C are always made with a high TEL level. As indicated in Table 4.7, each gasoline has two requirements: a maximum allowable Reid vapor pressure and a minimum required octane rating. In addition to these requirements, the company wants to ensure that the amount of gas A produced is at least as large as the amount of gas B produced.

Dave believes that Jansen can sell all of the gasoline it produces at the given prices. If any feedstocks are left over, they can be sold for the values indicated in Table 4.6. He wants to find a blending plan that meets all the requirements and maximizes the revenue from selling gasoline and leftover feedstocks. To help Dave with this problem, you should develop an LP optimization model and then use Solver to find the optimal blending plan. Then, using this model as a starting point, you should answer the following questions.

Table 4.6:

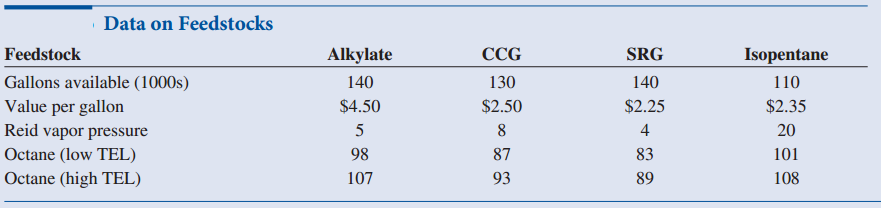
Table 4.7:

Transcribed Image Text:

Data on Feedstocks Feedstock Gallons available (1000s) Value per gallon Reid vapor pressure Octane (low TEL) Octane (high TEL) SRG Alkylate 140 CCG Isopentane 130 140 $2.25 4 110 $2.35 $2.50 $4.50 5 98 107 20 87 83 101 89 108 93 Data on Gasoline Gasoline Gallons required (1000s) Price per gallon Max Reid pressure Min octane TEL level A B 120 130 120 $3.00 $3.50 $4.00 90 97 100 Low High High