The relative growth rate r of a function f measures the rate of change of the function compared to its value at a particular point. It is computed as r(t) = ƒ'(t)/ƒ(t).a. Confirm that the relative growth rate in 1999 1t = 02 for the logistic model in Exercise 94 is r(0) = P'(0)/P(0) = 0.015. This means the world’s population was growing at 1.5% per year in 1999.b. Compute the relative growth rate of the world’s population in 2010 and 2020. What appears to be happening to the relative growth rate as time increases?

c. Evaluate 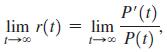where P(t) is the logistic growth function from Exercise 94. What does your answer say about populations that follow a logistic growth pattern?

Data From Exercise 3.8.94

The population of the world reached 6 billion in 1999 (t = 0). Assume Earth’s carrying capacity is 15 billion and the base growth rate is r₀ = 0.025 per year.

Transcribed Image Text:

P' (t) 1-8 P(t) lim r(t) = lim 1-0