A function f is called homogeneous of degree n if it satisfies the equation f (tx, ty)
Question:
A function f is called homogeneous of degree n if it satisfies the equation f (tx, ty) = tnf (x, y)
for all t, where n is a positive integer and f has continuous second-order partial derivatives.
(a) Verify that f (x, y) = x2y + 2xy2 + 5y3 is homogeneous of degree 3.
(b) Show that if f is homogeneous of degree n, then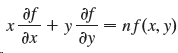
Transcribed Image Text:
af -nf(x, y) af + y* ду дх
Fantastic news! We've Found the answer you've been seeking!
Step by Step Answer:
Answer rating: 80% (15 reviews)
a Since f is a polynomial it has continuous secondorder partial derivatives an...View the full answer

Answered By

Ashington Waweru
I am a lecturer, research writer and also a qualified financial analyst and accountant. I am qualified and articulate in many disciplines including English, Accounting, Finance, Quantitative spreadsheet analysis, Economics, and Statistics. I am an expert with sixteen years of experience in online industry-related work. I have a master's in business administration and a bachelor’s degree in education, accounting, and economics options.
I am a writer and proofreading expert with sixteen years of experience in online writing, proofreading, and text editing. I have vast knowledge and experience in writing techniques and styles such as APA, ASA, MLA, Chicago, Turabian, IEEE, and many others.
I am also an online blogger and research writer with sixteen years of writing and proofreading articles and reports. I have written many scripts and articles for blogs, and I also specialize in search engine
I have sixteen years of experience in Excel data entry, Excel data analysis, R-studio quantitative analysis, SPSS quantitative analysis, research writing, and proofreading articles and reports. I will deliver the highest quality online and offline Excel, R, SPSS, and other spreadsheet solutions within your operational deadlines. I have also compiled many original Excel quantitative and text spreadsheets which solve client’s problems in my research writing career.
I have extensive enterprise resource planning accounting, financial modeling, financial reporting, and company analysis: customer relationship management, enterprise resource planning, financial accounting projects, and corporate finance.
I am articulate in psychology, engineering, nursing, counseling, project management, accounting, finance, quantitative spreadsheet analysis, statistical and economic analysis, among many other industry fields and academic disciplines. I work to solve problems and provide accurate and credible solutions and research reports in all industries in the global economy.
I have taught and conducted masters and Ph.D. thesis research for specialists in Quantitative finance, Financial Accounting, Actuarial science, Macroeconomics, Microeconomics, Risk Management, Managerial Economics, Engineering Economics, Financial economics, Taxation and many other disciplines including water engineering, psychology, e-commerce, mechanical engineering, leadership and many others.
I have developed many courses on online websites like Teachable and Thinkific. I also developed an accounting reporting automation software project for Utafiti sacco located at ILRI Uthiru Kenya when I was working there in year 2001.
I am a mature, self-motivated worker who delivers high-quality, on-time reports which solve client’s problems accurately.
I have written many academic and professional industry research papers and tutored many clients from college to university undergraduate, master's and Ph.D. students, and corporate professionals. I anticipate your hiring me.
I know I will deliver the highest quality work you will find anywhere to award me your project work. Please note that I am looking for a long-term work relationship with you. I look forward to you delivering the best service to you.
3.00+
2+ Reviews
10+ Question Solved
Related Book For 

Question Posted:
Students also viewed these Mathematics questions
-
A function f(x) on Rn is called homogeneous of degree k if f(cx) = ci(x) for all scalars c. (a) If a Rn is a fixed vector, show that a linear form (x) = a . x = a1X1 +-----+ anxn is homogeneous of...
-
If f is homogeneous of degree n, show that 'f ? 'f + y?- ,2 ? n(n 1)/(, ) + 2
-
Recall that a function f is called even if f(-x) = f(x) for all in its domain and odd if f(-x) = -f(x) for all such x. Prove each of the following. (a) The derivative of an even function is an odd...
-
Requirement 1. Journalize the partners' initial contributions. (Record debits first, then, credits. Select the explanation on the last line of the journal entry table.) Begin by journalizing the...
-
Heliy Company sells bonds that cost $40,000 for $45,000, including $1,000 of accrued interest. In recording the sale, Heliy books a $5,000 gain. Is this correct? Explain
-
Rewrite the following loops, using the enhanced for loop construct. Here, values is an array of floating-point numbers. a. for (int i = 0; i < values.length; i++) { total = total + values[i]; } b....
-
Critique several of the media sources you have identified using the following questions: (p. 56) A. Whose perspective is most prominently portrayed? B. What biases are evident? Why do you think they...
-
You are the manager of an organization in America that distributes blood to hospitals in all 50 states and the District of Columbia. Arecent report indicates that nearly 50 Americans contract HIV...
-
Calculate the average accounting return (AAR) using excel (make formulas viewable) . If you know that the required average accounting return is 25%. Would you accept that project? Calculate...
-
Arbortech, a designer, manufacturer, and marketer of PC cards for computers, printers, telecommunications equipment, and equipment diagnostic systems, was the darling of Wall Street during Year 6....
-
Assume that all the given functions have continuous second-order partial derivatives. Suppose z = f (x, y), where x = g(s, t) and y = h(s, t). (a) Show that (b) Find a similar formula for 2z/s t. ?z ...
-
If f is homogeneous of degree n, show that f x (tx, ty) = t n-1 f x (x, y)
-
Veronica Mars, a recent graduate of Bells accounting program, evaluated the operating performance of Dunn Companys six divisions. Veronica made the following presentation to Dunns board of directors...
-
In countries with high unemployment and poverty rates, the nation's people are often more concerned with the economic environment than the intricacies of its political systems. In 2010, Mohamed...
-
Analyze this approach: Consider that you could increase the productivity of your department, you have thought about certain ways to do it, but you are not sure. Your team has a lot of experience, but...
-
What is the research question or objective? What research methods did the authors use? Examples include survey, case study, interviews, opinions, qualitative, quantitative, etc. What are the...
-
Provide a critical reflection on each department outlining which services, aspects and operational factors you should further investigate to help improve customer satisfaction. Express the negative...
-
1. Many courses use group projects. What are some of the things that make positive group project experiences? 2. How can a manager motivate employees? Give some specific ideas. Include when you've...
-
How many daily rainfall amounts in Boston must be randomly selected to estimate the mean daily rainfall amount? We want 99% confidence that the sample mean is within 0.010 in. of the population mean,...
-
Multiple Choice Questions: 1. The largest component of aggregate demand is? a. Government purchases. b. Net exports. c. Consumption. d. Investment. 2. A reduction in personal income taxes, other...
-
Suppose that circles of equal diameter are packed tightly in rows inside an equilateral triangle. (The figure illustrates the case n = 4.) If A is the area of the triangle and An is the total area...
-
Taking the value of xx at 0 to be 1 and integrating a series term by term, show that 1n
-
Find the sum of the series (- 1)n / (2n + 1)3n
-
How do external factors such as changing consumer preferences affect the retail industry?"
-
Production costs that are not attached to units that are sold are reported as: Cost of goods sold Selling expenses Administrative costs Inventory
-
Please show workings :) Oxford Company has limited funds available for investment and must ration the funds among four competing projects. Selected information on the four projects follows: Life of...

Study smarter with the SolutionInn App


