a. Let a = x 0 < x 1 < x 2 < x
Question:
a. Let a = x0 < x1 < x2 · · · < xn = b be any partition of [a, b], and let F be any antiderivative of ƒ. Show that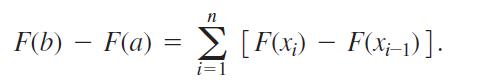
b. Apply the Mean Value Theorem to each term to show that F(xi) - F(xi-1) = ƒ(ci)(xi - xi-1) for some ci in the interval (xi-1, xi). Then show that F(b) - F(a) is a Riemann sum for ƒ on [a, b].
c. From part (b) and the definition of the definite integral, show that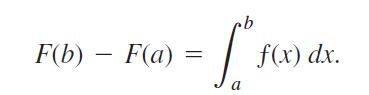
Fantastic news! We've Found the answer you've been seeking!
Step by Step Answer:
Related Book For 

Thomas Calculus Early Transcendentals
ISBN: 9780321884077
13th Edition
Authors: Joel R Hass, Christopher E Heil, Maurice D Weir
Question Posted:





