Suppose that the parametrized curve C: ((u), g(u)) is revolved about the x-axis, where g(u) > 0 for a u b. a. Show

Suppose that the parametrized curve C: (ƒ(u), g(u)) is revolved about the x-axis, where g(u) > 0 for a ≤ u ≤ b.

a. Show that

is a parametrization of the resulting surface of revolution, where 0 ≤ ν ≤ 2π is the angle from the xy-plane to the point r(u, ν) on the surface. Notice that ƒ(u) measures distance along the axis of revolution and g(u) measures distance from the axis of revolution.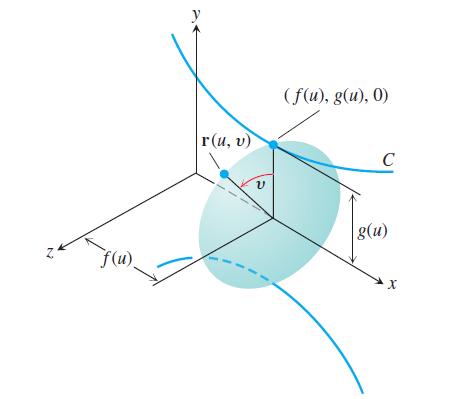

b. Find a parametrization for the surface obtained by revolving the curve x = y², y ≥ 0, about the x-axis.

r(u, v) = f(u)i + (g(u)cos v)j + (g(u)sin v)k