Two Gaussian random variables X and Y, with zero means and variances Ï 2 , between which

Question:

Two Gaussian random variables X and Y, with zero means and variances Ïƒ², between which there is a correlation coefficient p, have a joint probability-density function given by

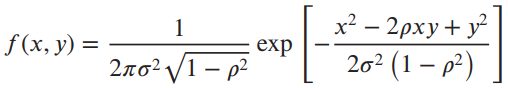
x² – 2pxy+ y f(x, y) = exp 2πσ2 V1 -ρ2 202 (1 – p²)

The marginal pdf of Y can be shown to be

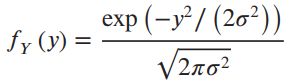
exp (-y²/ (2o²)) fy (y) = V2ло?

Find the conditional pdf f_X|Y(X | Y).

Fantastic news! We've Found the answer you've been seeking!

Step by Step Answer:

Related Book For book-img-for-question

Principles of Communications Systems, Modulation and Noise

ISBN: 978-8126556793

7th edition

Authors: Rodger E. Ziemer, William H. Tranter

See More Books

Question Posted: Sep 19, 2018 01:09 PM

See More Questions

Two Gaussian random variables, X and Y, are in dependent. Their respective means are 5 and 3, and their respective variances are 1 and 2. (a) Write down expressions for their marginal pdfs. (b) Write...
Two Gaussian random variables, X and Y, are in dependent. Their respective means are 4 and 2, and their respective variances are 3 and 5. (a) Write down expressions for their marginal pdfs. (b) Write...
Two jointly Gaussian random variables, X and Y, have means of 1 and 2, and variances of 3 and 2, respectively. Their correlation coefficient is P xy = 0.2. A new random variable is defined as Z = 3X...
For rigid-body motion, the strains will vanish. Under these conditions, integrate the straindisplacement relations (7.6.1) to show that the most general form of a rigid-body motion displacement field...
The head of the United Kumquat Growers Association makes the following statement: The federal government is considering implementing a price floor in the market for kumquats. The government will not...
Discuss the two dimensions of environmental uncertainty. LO5
What is the difference between the international monetary system and the global financial system? LO.1
Here is financial information for Pauletti Inc. InstructionsPrepare a schedule showing a horizontal analysis for 2012, using 2011 as the base year. December 31, 2012 December 31, 2011 Current assets...
Code Section __1_ spells out the gross income for landlords (lessor) when they have improvements made by their tenants (lessee) on the rental property. Code Section __2__ is about the tax basis of...
Explain the entrepreneurial characteristics of Tariq. How did they evolve over a period of time? Tariq a Mechanical Engineer graduated from University of Engineering and Technology. After which he...
Two random variables X and Y have means and variances given below: m x = 1 2 x = 4 m y = 3 2 y = 7 A new random variable Z is defined as Z = 3X - 4Y Determine the mean and variance of Z for each of...
Using the definition of a conditional pdf given by Equation (6.62) and the expressions for the marginal and joint Gaussian pdfs, show that for two jointly Gaussian random variables X and Y, the...
For Problem write in simpler form, as in Example 4. log b FG Example 4 For f(x) = 12/ (x - 2), g(x) = 1 - x 2 , and h(x) = x - 1, evaluate: (A) f(6) (B) g(-2) (C) h(-2) (D) f(0) + g(1) - h(10)
Match each of the following transactions of Lesch \& Company with the appropriate letters, indicating the debits and credits to be made. The key for the letters follows the list of transactions. The...
Workers act as sellers of their time in the labor market in return for some wage. Lets discover your individual supply curve for labor. For each hourly wage rate provided in the accompanying table,...
The Joint Commission on Accreditation of Healthcare Organizations (JCAHO) monitors and evaluates health care providers according to strict standards and guidelines. Improvement in the quality of care...
The U.S. National Highway Traffic Safety Administratio (NHTSA) independently tests over 2,400 types of tires annually. In 2015, they issued more than 900 recalls, affecting 51 million vehicles...
The Hudson Jewelers case study can be found in Appendix C. Chapter 17 Case Questions for Discussion: 1.Research and acquire the criteria for diamond appraisals and critique these criteria in terms of...
Use graphing software to determine which of the given viewing windows displays the most appropriate graph of the specified function. (x) = x 3 - 4x 2 - 4x + 16 a. [-1, 1] by [-5, 5] b. [-3, 3] by...
If there is an unrealized holding gain on available-for-sale investments, it is reported as?
A particles velocity is described by the function v x = (t 2 - 7t + 10) m/s, where t is in s. a. At what times does the particle reach its turning points? b. What is the particles acceleration at...
A particles velocity is described by the function v x = k t 2 m/s, where k is a constant and t is in s. The particles position at t 0 = 0 s is x 0 = - 9.0 m. At t 1 = 3.0 s, the particle is at x 1 =...
A particles acceleration is described by the function a x = (10 t) m/s 2 , where t is in s. Its initial conditions are x 0 = 0 m and v 0x = 0 m/s at t = 0 s. a. At what time is the velocity again...
1. Determine the value of the right to use asset and lease liability at commencement of the lease.
Problem 22-1 The management of Sunland Instrument Company had concluded, with the concurrence of its independent auditors, that results of operations would be more fairly presented if Sunland changed...
Question 4. - Week 9. What are the major competitive issues General Electric faces when managing cooperative strategies? - (7 marks)

Two Gaussian random variables X and Y, with zero means and variances Ï 2 , between which

Question:

x² – 2pxy+ y f(x, y) = exp 2πσ2 V1 -ρ2 202 (1 – p²) exp (-y²/ (2o²)) fy (y) = V2ло?

Step by Step Answer:

The conditional pdf f XY xy is given by Note that the conditional pdf of X given Y is Gaussian ...View the full answer

Principles of Communications Systems, Modulation and Noise

Students also viewed these Physics questions