a. Determine the allowable torque, (T), if the crack length for the shaft in Figure 9.8 is
Question:
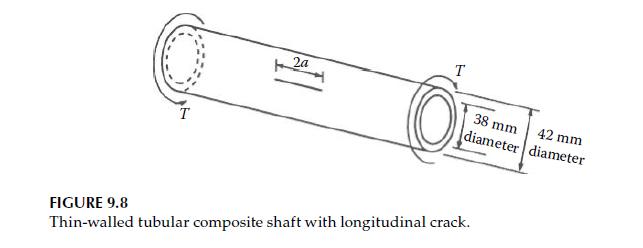
a. Determine the allowable torque, \(T\), if the crack length for the shaft in Figure 9.8 is \(2 a=10 \mathrm{~mm}\). Use the same dimensions and fracture toughness values that were given in Problem 9.2.
b. If the uniaxial yield stress for the shaft material is \(Y=1200 \mathrm{MPa}\), and the crack is ignored, compare the answer from part (a) with the allowable torque based on the maximum shear stress criterion for yielding.
Problem 9.2
The thin-walled tubular shaft shown in Figure 9.8 is made of a randomly oriented, short-fiber-reinforced metal matrix composite. The shaft has a longitudinal through-thickness crack of length \(2 a\) and is subjected to a torque \(T=1 \mathrm{KN} \mathrm{m}\). If the mode II fracture toughness of the composite is \(K_{\text {IIc }}=40 \mathrm{MPa} \mathrm{m}^{1 / 2}\), determine the critical crack size for self-sustaining crack growth.
Step by Step Answer:

Principles Of Composite Material Mechanics
ISBN: 9781498720694
4th Edition
Authors: Ronald F. Gibson





