A thin-walled cylindrical pressure vessel has mean diameter \(d=18\) in. and wall thickness \(h=0.25\) in. The vessel is made of filament-wound unidirectional composite material with all fibers oriented in the circumferential, or hoop, direction. The internal pressure in the vessel can be assumed to be constant. From creep tests of specimens of the unidirectional composite material, it is found that the principal creep compliances can be described by the following power-law expressions:

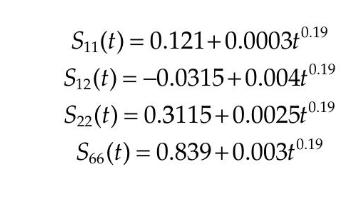
where \(t\) is the time in minutes and all compliances are given in units of \(\left(\times 10^{-6}\right.\) \(\mathrm{psi}^{-1}\) ). The ultimate failure strains for the material are found to be as follows:
\[\mathrm{e}_{\mathrm{L}}^{(+)}=0.0194, \quad \mathrm{e}_{\mathrm{T}}^{(+)}=0.00125, \quad \mathrm{e}_{\mathrm{LT}}=0.010\]

a. According to the maximum strain criterion, what is the allowable internal pressure in the vessel, if it is to be designed to last for at least 20 years under constant pressure?

b. How would you change the design of the vessel so as to increase the allowable internal pressure, while maintaining the 20-year design life?

Transcribed Image Text:

S1(t)=0.121+0.00031 0.19 S12(t)=-0.0315+0.004+0.19 S22(t)=0.3115+0.0025+0.19 S66(t)=0.839+0.003+0.19