4.4. This problem considers a continuous time Markov chain model for the changing pattern of relationships among members in a group. The group has four members:

a, b,

c, and

d. Each pair of the group may or may not have a certain relationship with each other. If they have the relationship, we say that they are linked. For example, being linked may mean that the two members are communicating with each other. The following graph illustrates links between a and

b, between a and

c, and between b and d:

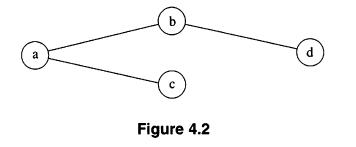
Suppose that any pair of unlinked individuals will become linked in a small time interval of length h with probability ah + o(h). Any pair of linked individuals will lose their link in a small time interval of length h with probability /3h + o(h). Let X(t) denote the number of linked pairs of individuals in the group at time t. Then X(t) is a birth and death process.

(a) Specify the birth and death parameters Ak and /j,, for k = 0, 1.... .

(b) Determine the stationary distribution for the process.

Transcribed Image Text:

a b d Figure 4.2