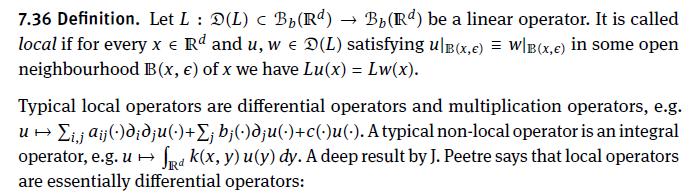
Assume that (L: mathcal{C}_{c}^{infty}left(mathbb{R}^{d} ight) ightarrow mathcal{C}left(mathbb{R}^{d} ight)) is a local operator (cf. Definition 7.36) which is almost positive, i.e. for all (phi in

Assume that \(L: \mathcal{C}_{c}^{\infty}\left(\mathbb{R}^{d}\right) \rightarrow \mathcal{C}\left(\mathbb{R}^{d}\right)\) is a local operator (cf. Definition 7.36) which is almost positive, i.e. for all \(\phi \in \mathcal{C}_{c}^{\infty}\) such that \(\phi \geqslant 0\) and \(\phi\left(x_{0}\right)=0\) we have \(L \phi\left(x_{0}\right) \geqslant 0\).

a) Show that an operator that satisfies the positive maximum principle is almost positive. (This does not require locality).

b) Show that \(\operatorname{supp} L \phi \subset \operatorname{supp} \phi\).

c) Show that the operator \(L\) is of order two, i.e. \(\|L \phi\|_{\infty} \leqslant C\|\phi\|_{(2)}\) holds for all \(\phi \in \mathcal{C}_{c}^{\infty}(K)\) and any compact set \(K \subset \mathbb{R}^{d}\). Here, \(\|\phi\|_{(2)}=\sum_{0 \leqslant|\alpha| \leqslant 2}\left\|\partial^{\alpha} \phi\right\|_{\infty}\) and the constant \(C\) depends only on \(K\).

Consider \(f(y):=c\|\phi\|_{(2)}|x-y|^{2} \chi(y) \pm(\phi(y)-\phi(x) \chi(y)-abla \phi(x) \cdot(y-x) \chi(y))\) where \(\mathbb{1}_{K} \leqslant \chi \leqslant 1\) is a smooth cut-off function and \(c\) is a sufficiently large constant. Now apply almost positivity at \(x_{0}=x\).

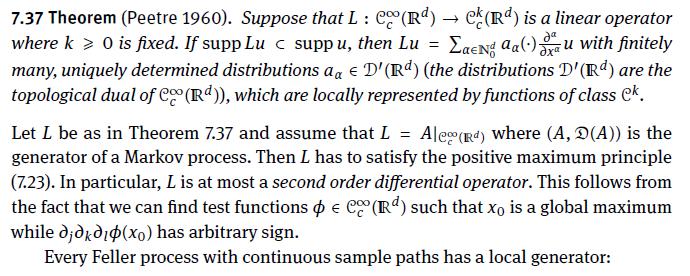
d) Use Theorem 7.37 to conclude that \(L\) is a differential operator of order \(\leqslant 2\).

Data From Definition 7.36

Data From Theorem 7.37

t -> 7.36 Definition. Let L: (L) c B(Rd). B(Rd) be a linear operator. It is called local if for every xe Rd and u, w = Q(L) satisfying u|B(x,e) = W|B(x,e) in some open neighbourhood B(x, e) of x we have Lu(x) = Lw(x). Typical local operators are differential operators and multiplication operators, e.g. u ij ()didu()+; b;()dju()+c()u(). A typical non-local operator is an integral operator, e.g. u fd k(x, y) u(y) dy. A deep result by J. Peetre says that local operators are essentially differential operators: