Let (f) be the initial value in the problem considered in Lemma 8.1 and write (u(t, x):=) (P_{t} f(x)=mathbb{E} fleft(B_{t}+x ight)) where (left(B_{t} ight)_{t geqslant

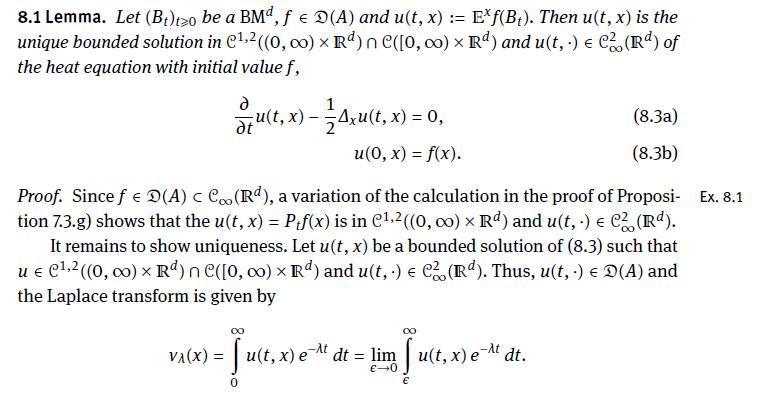
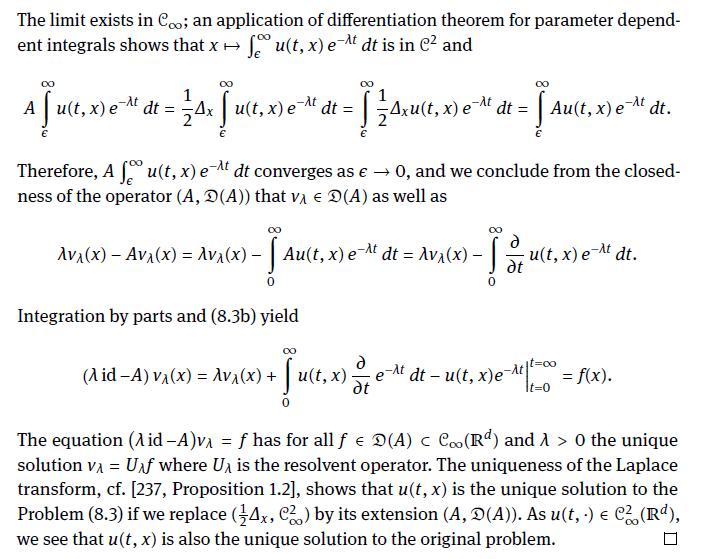
Let \(f\) be the initial value in the problem considered in Lemma 8.1 and write \(u(t, x):=\) \(P_{t} f(x)=\mathbb{E} f\left(B_{t}+x\right)\) where \(\left(B_{t}\right)_{t \geqslant 0}\) is a BM \({ }^{d}\).

a) Assume that \(f \in \mathcal{C}_{\infty}\left(\mathbb{R}^{d}\right)\). Show that \(\partial_{t} u(t, x), \partial_{j} u(t, x)\) and \(\partial_{j} \partial_{k} u(t, x)\) exist and are functions in \(\mathcal{C}\left((0, \infty) \times \mathbb{R}^{d}\right)\).

b) Assume that \(f: \mathbb{R}^{d} \rightarrow \mathbb{R}\) is measurable and exponentially bounded, i.e. \(|f(x)| \leqslant C e^{C|x|}\) for all \(x \in \mathbb{R}^{d}\) and some constant \(C\). Show that \(\partial_{t} u(t, x), \partial_{j} u(t, x)\) and \(\partial_{j} \partial_{k} u(t, x), j, k=1, \ldots, d\), exist and are exponentially bounded functions in \(\mathcal{C}\left((0, \infty) \times \mathbb{R}^{d}\right)\).

c) What happens, if we assume in Part b) that \(f(x) \leqslant C e^{C|x|^{2}}\) ?

Data From  Lemma 8.1

8.1 Lemma. Let (Bt)to be a BMd, f = D(A) and u(t, x) = Exf(B+). Then u(t, x) is the unique bounded solution in e.2 ((0,00) x Rd) n C([0, 0) x Rd) and u(t,) e C (Rd) of the heat equation with initial value f, ru(t, x) - Axu(t, x) = 0, u(0, x) = f(x). (8.3a) (8.3b) Proof. Since f = D(A) c Co. (Rd), a variation of the calculation in the proof of Proposi- Ex. 8.1 tion 7.3.g) shows that the u(t, x) = Pf(x) is in e.2 ((0, 0) x Rd) and u(t,) C (Rd). It remains to show uniqueness. Let u(t, x) be a bounded solution of (8.3) such that u 1,2((0,00) x Rd) ne([0, 00) x Rd) and u(t,) 2 (Rd). Thus, u(t,) = D(A) and the Laplace transform is given by VA(x) = u(t, x) e- dt = lim u(t, x) e dt. 0