Let (B=left(B_{t} ight)_{t geqslant 0}) be a canonical (mathrm{BM}^{1}) on Wiener space ((Omega, mathscr{A}, mathbb{P})=left(mathcal{C}_{(0)}, mathscr{B}left(mathcal{C}_{(0)} ight),

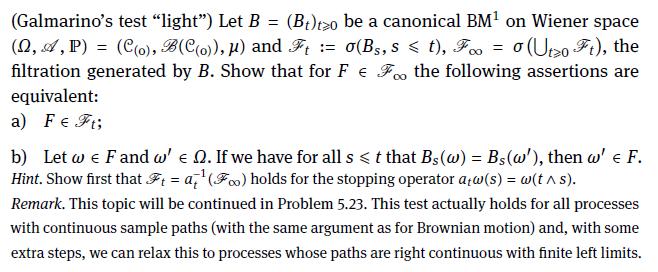
Let \(B=\left(B_{t}\right)_{t \geqslant 0}\) be a canonical \(\mathrm{BM}^{1}\) on Wiener space \((\Omega, \mathscr{A}, \mathbb{P})=\left(\mathcal{C}_{(0)}, \mathscr{B}\left(\mathcal{C}_{(0)}\right), \mu\right)\) and \(\mathscr{F}_{t}:=\sigma\left(B_{s}, s \leqslant t\right), \mathscr{F}_{\infty}=\sigma\left(\bigcup_{t \geqslant 0} \mathscr{F}_{t}\right)\), the filtration generated by \(B\). Show that for \(F \in \mathscr{F}_{\infty}\) the following assertions are equivalent:

a) \(F \in \mathscr{F}_{t}\);

b) Let \(\omega \in F\) and \(\omega^{\prime} \in \Omega\). If we have for all \(s \leqslant t\) that \(B_{s}(\omega)=B_{s}\left(\omega^{\prime}\right)\), then \(\omega^{\prime} \in F\).Show first that \(\mathscr{F}_{t}=a_{t}^{-1}\left(\mathscr{F}_{\infty}\right)\) holds for the stopping operator \(a_{t} \omega(s)=\omega(t \wedge s)\).

Remark. This topic will be continued in Problem 5.23. This test actually holds for all processes with continuous sample paths (with the same argument as for Brownian motion) and, with some extra steps, we can relax this to processes whose paths are right continuous with finite left limits.

Data From  Problem 5.23

Transcribed Image Text:

(Galmarino's test "light") Let B = (Bt)to be a canonical BM on Wiener space (,, P) (C(0), B(C(o)), ) and Ft := (Bs, s < t), F = (Ut20 Ft), the filtration generated by B. Show that for F = Foo the following assertions are equivalent: a) F = Ft; b) Let w F and w' 2. If we have for all s