We continue with the set-up introduced in Problem 7.Denote by (left(mathcal{C}_{(0)}, mathscr{B}left(mathcal{C}_{(0)} ight), mu ight)) the canonical
Question:
We continue with the set-up introduced in Problem 7.Denote by \(\left(\mathcal{C}_{(0)}, \mathscr{B}\left(\mathcal{C}_{(0)}\right), \mu\right)\) the canonical Wiener space and set \(\Omega=\mathcal{C}, \mathscr{A}=\mathscr{B}(\mathcal{C})\), \(B_{t}(w)=w(t)\) for \(w \in \Omega\), and define for every probability measure \(\pi\) on \((\mathbb{R}, \mathscr{B}(\mathbb{R}))\) a probability measure \(\mathbb{P}^{\pi}\) on \((\Omega, \mathscr{B}(\mathcal{C})\) ) first on the "cylinder sets"\[\begin{aligned}& \mathbb{P}^{\pi}\left(\left\{w: w\left(t_{0}\right) \in C_{0}, w\left(t_{1}\right) \in C_{1}, \ldots, w\left(t_{n}\right) \in C_{n}\right\}\right) \\& \quad:=\int_{C_{0}} \mu\left(\left\{w: w\left(t_{1}\right)+x \in C_{1}, \ldots, w\left(t_{n}\right)+x \in C_{n}\right\}\right) \pi(d x),\end{aligned}\]
where \(n \in \mathbb{N}_{0}, t_{0}=0 a) Show that the map \(w \mapsto B(\cdot, w)=\left\{t \mapsto B_{t}(w)\right\}\) is measurable. b) Show that \(B_{t}\) is, under \(\mathbb{P}^{\pi}\) and \(\pi=\delta_{x}\), a Brownian motion started in \(B_{0}=x\), i.e. a process satisfying (B1), (B2), (B4) and \(B_{0}=x\) instead of (B0) and \(B_{t} \sim \mathrm{N}(x, t)\) instead of (B3). c) Give an interpretation as to what \(\mathbb{P}^{\pi}\) means at the level of paths. Data From Theorem 4.8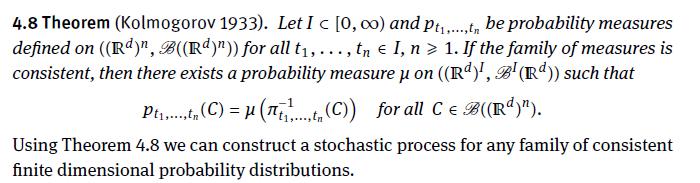
Step by Step Answer:

Brownian Motion A Guide To Random Processes And Stochastic Calculus De Gruyter Textbook
ISBN: 9783110741254
3rd Edition
Authors: René L. Schilling, Björn Böttcher





