Let (g:[0, infty) times mathbb{R}^{d} ightarrow mathbb{R}) be a bounded continuous function such that (g(t, cdot))

Let \(g:[0, \infty) \times \mathbb{R}^{d} \rightarrow \mathbb{R}\) be a bounded continuous function such that \(g(t, \cdot)\) is \(\kappa\)-Hölder continuous with a Hölder constant which does not depend on \(t\) or \(x\). Mimic the proof of Lemma 8.7 to prove that \(v(t, x):=\mathbb{E}^{x}\left(\int_{0}^{t} g\left(t-s, B_{s}\right) d s\right)\) is in \(\mathcal{C}^{1,2}\left((0, \infty) \times \mathbb{R}^{d}\right) \cap \mathcal{C}_{b}\left([0, \infty) \times \mathbb{R}^{d}\right)\) and satisfies (8.1). Explain why this proves, under suitable assumptions on \(g(t, x)\), No.3 in Table 8.1.

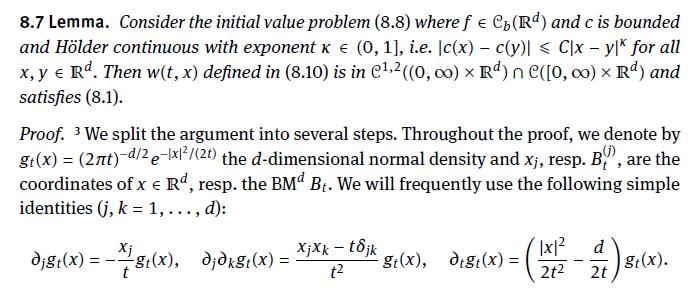
Data From Lemma 8.7

Transcribed Image Text:

- 8.7 Lemma. Consider the initial value problem (8.8) where f = C(Rd) and c is bounded and Hlder continuous with exponent = (0, 1], i.e. |c(x) - c(y)| C|x y|* for all x, y = Rd. Then w(t, x) defined in (8.10) is in e,2 ((0,00) x Rd) n C([0, 0) Rd) and satisfies (8.1). Proof. 3 We split the argument into several steps. Throughout the proof, we denote by gt(x) = (2t)-d/2e-x/(2t) the d-dimensional normal density and x;, resp. B", are the coordinates of x = Rd, resp. the BMd Bt. We will frequently use the following simple identities (j, k = 1,..., d): Xj digt(x) = () == -1(x), g(x), djdkgt(x) xjxk-tjk gt(x), digt (x) = t2 (1/1/2 d gt(x). 2t