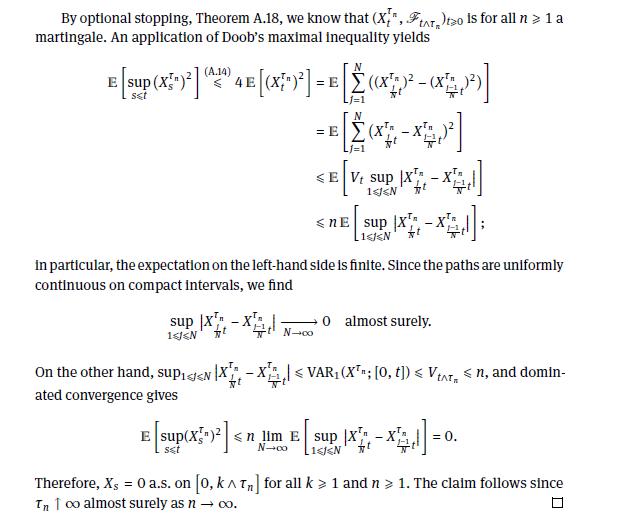
Use the fact that any continuous, square-integrable martingale with bounded variation paths is constant (cf. Proposition 17.2)
Question:
Use the fact that any continuous, square-integrable martingale with bounded variation paths is constant (cf. Proposition 17.2) to show the following: \(\langle f \cdot Bangle_{t}:=\int_{0}^{t}|f(s)|^{2} d s\) is the unique continuous and increasing process such that \((f \cdot B)^{2}-f^{2} \cdot\langle Bangle\) is a martingale.
Data From Proposition 17.2
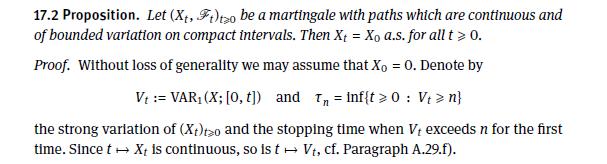
Fantastic news! We've Found the answer you've been seeking!
Step by Step Answer:
Related Book For 

Brownian Motion A Guide To Random Processes And Stochastic Calculus De Gruyter Textbook
ISBN: 9783110741254
3rd Edition
Authors: René L. Schilling, Björn Böttcher
Question Posted:





