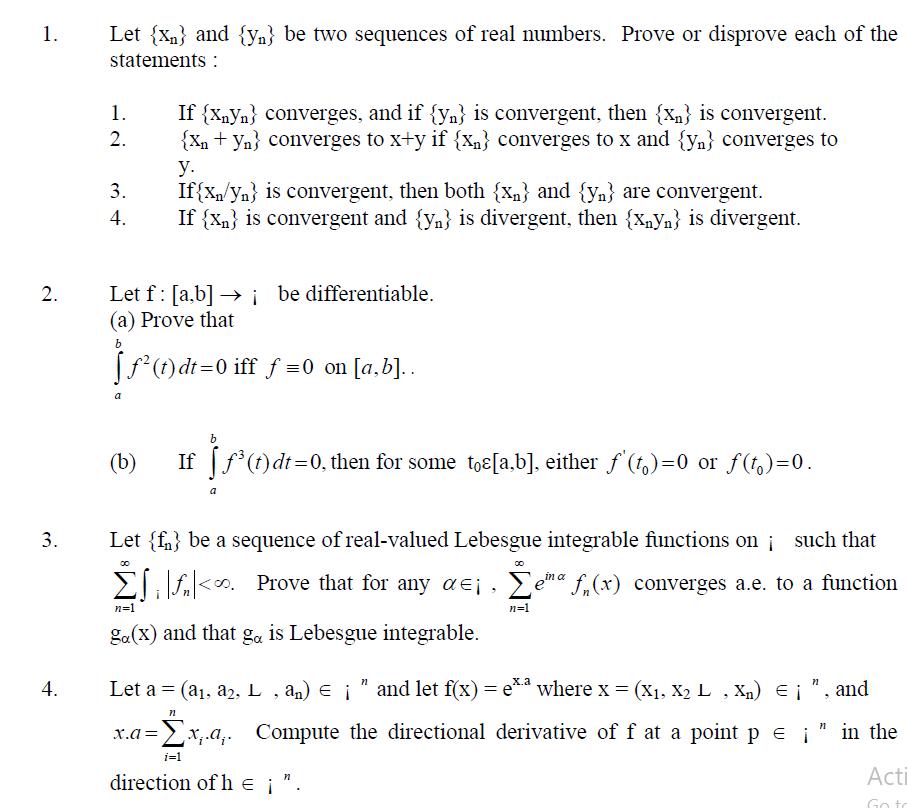
1. 2. 3. 4. Let {x} and {y} be two sequences of real numbers. Prove or disprove each of the statements: 1. 12 2. 3. 34 4. If {xnyn} converges, and if {y} is convergent, then {x} is convergent. {Xn+yn} converges to x+y if {n} converges to x and {yn} converges to y. If{xn/yn} is convergent, then both {x} and {yn} are convergent. If {x} is convergent and {y} is divergent, then {xyn} is divergent. Let f [a,b] be differentiable. (a) Prove that b [f(t)dt=0 iff f=0 on [a,b]. a (b) b If f (t) dt=0, then for some to[a,b], either *(t)=0 or f(t)=0. a Let {f} be a sequence of real-valued Lebesgue integrable functions on such that 00 00 . , < Prove that for any =j. e in a f(x) converges a.e. to a function n=1 ga(x) and that ga is Lebesgue integrable. == n=1 Let a = (a1, a2, L, an) E " and let f(x) = exa where x = (X1, X2 L, X) E ", and n x.ax.a. Compute the directional derivative of f at a point pe " in the i=1 direction of he". Acti Go to