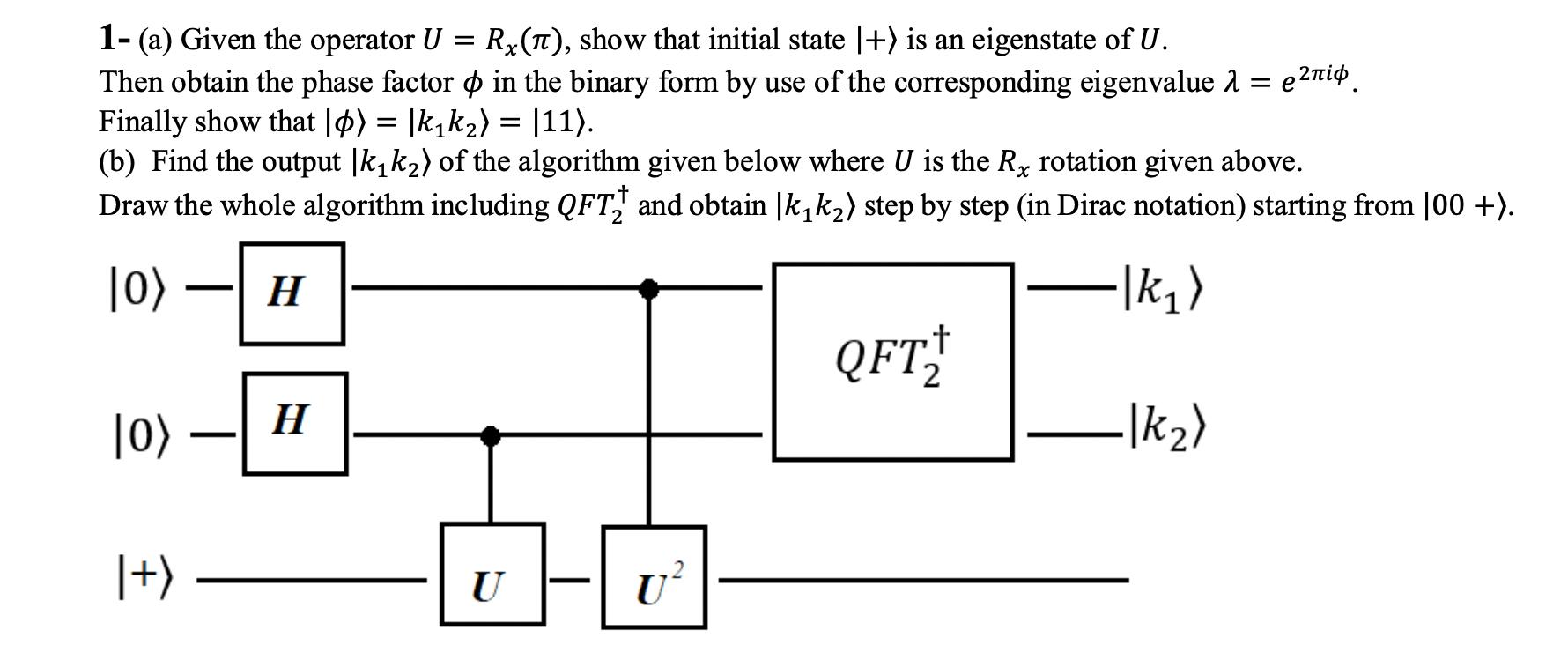
1- (a) Given the operator U = Rx(T), show that initial state (+) is an eigenstate of U. Then obtain the phase factor in the binary form by use of the corresponding eigenvalue = ei. Finally show that |0) = |kk2) = |11). (b) Find the output |kk2) of the algorithm given below where U is the Rx rotation given above. Draw the whole algorithm including QFT and obtain |kk2) step by step (in Dirac notation) starting from |00 +). |0) H F |+) - H U - U |k) QFT |k)