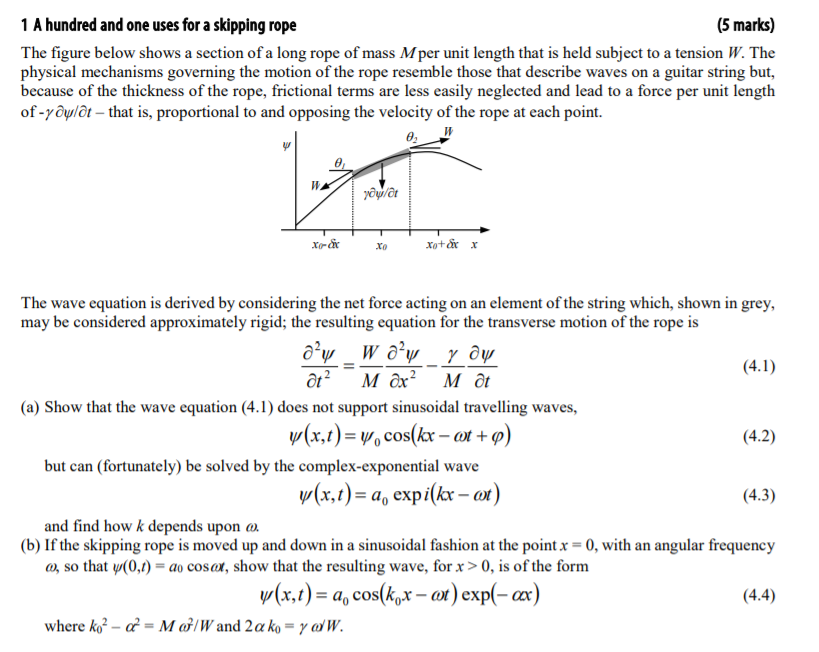
1 A hundred and one uses for a skipping rope (5 marks) The figure below shows a section of a long rope of mass M per unit length that is held subject to a tension W. The physical mechanisms governing the motion of the rope resemble those that describe waves on a guitar string but, because of the thickness of the rope, frictional terms are less easily neglected and lead to a force per unit length of-y@ylot - that is, proportional to and opposing the velocity of the rope at each point. plat Xord XO Xo+& X The wave equation is derived by considering the net force acting on an element of the string which, shown in grey, may be considered approximately rigid; the resulting equation for the transverse motion of the rope is ay _w ay y Oy (4.1) @t? M Ox? Mt (a) Show that the wave equation (4.1) does not support sinusoidal travelling waves, v(x,t)=v, cos(kx mt+ +0) (4.2) but can (fortunately) be solved by the complex-exponential wave v(x,t)= a, expi(kx wt) (4.3) and find how k depends upon o. (b) If the skipping rope is moved up and down in a sinusoidal fashion at the point x = 0, with an angular frequency @, so that y(0,1)= ao cosor, show that the resulting wave, for x > 0, is of the form v(x,t)= a, cos(kx ot) exp(-x) where ko? - ?- M o/Wand 2 a ko = y oW. (4.4) 1 A hundred and one uses for a skipping rope (5 marks) The figure below shows a section of a long rope of mass M per unit length that is held subject to a tension W. The physical mechanisms governing the motion of the rope resemble those that describe waves on a guitar string but, because of the thickness of the rope, frictional terms are less easily neglected and lead to a force per unit length of-y@ylot - that is, proportional to and opposing the velocity of the rope at each point. plat Xord XO Xo+& X The wave equation is derived by considering the net force acting on an element of the string which, shown in grey, may be considered approximately rigid; the resulting equation for the transverse motion of the rope is ay _w ay y Oy (4.1) @t? M Ox? Mt (a) Show that the wave equation (4.1) does not support sinusoidal travelling waves, v(x,t)=v, cos(kx mt+ +0) (4.2) but can (fortunately) be solved by the complex-exponential wave v(x,t)= a, expi(kx wt) (4.3) and find how k depends upon o. (b) If the skipping rope is moved up and down in a sinusoidal fashion at the point x = 0, with an angular frequency @, so that y(0,1)= ao cosor, show that the resulting wave, for x > 0, is of the form v(x,t)= a, cos(kx ot) exp(-x) where ko? - ?- M o/Wand 2 a ko = y oW. (4.4)