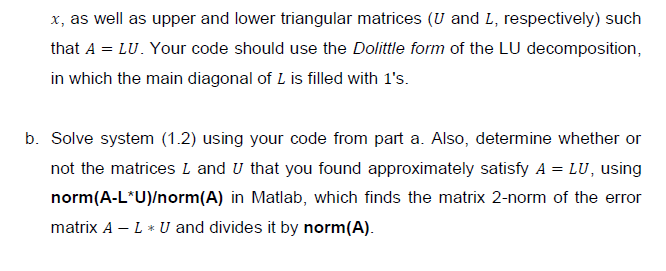
1. A set of experiments has found that the specific volume v of saturated steam in m3/Kg at six dimensionless temperature T values of 1, 2, 3, 4, 5, and 6 (where 1 represents 10C in physical units) is respectively 106.4, 57.79, 32.9, 19.52, 12.03, and 7.67. A fifth-degree polynomial of the form can be used to represent volume v as a function of temperature T. The unknown coefficients x1, x2, X3. x4 x5, and xs can be found by solving the following system of linear algebraic equations obtained by substituting the given data in equation (1.1): X1 X2 + X3 + X4 + X5 + X6-106.4 X1 + 2X2 + 23x3 + 23x4 + 24x5 + 2% = 57.7 x13x2 32x3 + 33x4+3x5 36 32.9 x, + 4x2 + 42x3 + 43x4 +44x5 + 45x6 19.52 x 5x2 52x3 + 53x4 54x5 55x6 12.03 X1 + 6X2 62X3 63X4 + 64X5 + 65X6-7.67 a. Write your own Matlab code that will solve an arbitrary n-dimensional system of linear equations, Ax=b, using the LU decomposition with standard Gaussian elimination without pivoting. Your code should take an arbitrary n n matrix A and n 1 vector b as inputs, and it should return the solution 1. A set of experiments has found that the specific volume v of saturated steam in m3/Kg at six dimensionless temperature T values of 1, 2, 3, 4, 5, and 6 (where 1 represents 10C in physical units) is respectively 106.4, 57.79, 32.9, 19.52, 12.03, and 7.67. A fifth-degree polynomial of the form can be used to represent volume v as a function of temperature T. The unknown coefficients x1, x2, X3. x4 x5, and xs can be found by solving the following system of linear algebraic equations obtained by substituting the given data in equation (1.1): X1 X2 + X3 + X4 + X5 + X6-106.4 X1 + 2X2 + 23x3 + 23x4 + 24x5 + 2% = 57.7 x13x2 32x3 + 33x4+3x5 36 32.9 x, + 4x2 + 42x3 + 43x4 +44x5 + 45x6 19.52 x 5x2 52x3 + 53x4 54x5 55x6 12.03 X1 + 6X2 62X3 63X4 + 64X5 + 65X6-7.67 a. Write your own Matlab code that will solve an arbitrary n-dimensional system of linear equations, Ax=b, using the LU decomposition with standard Gaussian elimination without pivoting. Your code should take an arbitrary n n matrix A and n 1 vector b as inputs, and it should return the solution