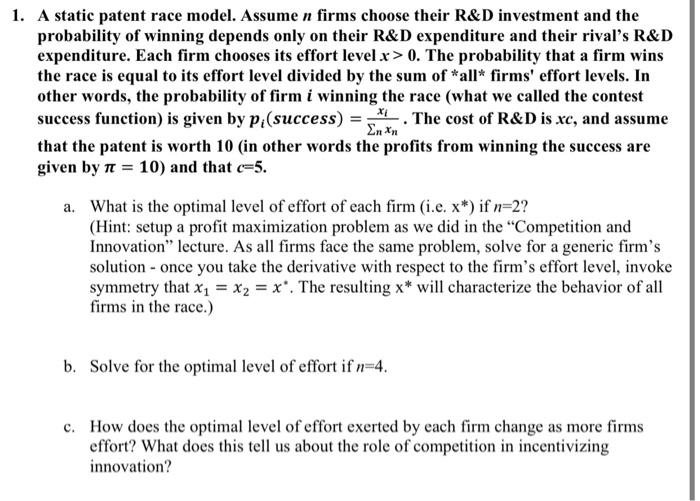
1. A static patent race model. Assume n firms choose their R&D investment and the probability of winning depends only on their R&D expenditure and their rival's R&D expenditure. Each firm chooses its effort level x > 0. The probability that a firm wins the race is equal to its effort level divided by the sum of *all* firms' effort levels. In other words, the probability of firm i winning the race (what we called the contest success function) is given by P;(success) = *. The cost of R&D is xc, and assume that the patent is worth 10 (in other words the profits from winning the success are given by n = 10) and that c=5. a. What is the optimal level of effort of each firm (i.e. X*) if n=2? (Hint: setup a profit maximization problem as we did in the Competition and Innovation" lecture. As all firms face the same problem, solve for a generic firm's solution - once you take the derivative with respect to the firm's effort level, invoke symmetry that x1 = x2 = x'. The resulting x* will characterize the behavior of all firms in the race.) b. Solve for the optimal level of effort if n=4. c. How does the optimal level of effort exerted by each firm change as more firms effort? What does this tell us about the role of competition in incentivizing innovation? 1. A static patent race model. Assume n firms choose their R&D investment and the probability of winning depends only on their R&D expenditure and their rival's R&D expenditure. Each firm chooses its effort level x > 0. The probability that a firm wins the race is equal to its effort level divided by the sum of *all* firms' effort levels. In other words, the probability of firm i winning the race (what we called the contest success function) is given by P;(success) = *. The cost of R&D is xc, and assume that the patent is worth 10 (in other words the profits from winning the success are given by n = 10) and that c=5. a. What is the optimal level of effort of each firm (i.e. X*) if n=2? (Hint: setup a profit maximization problem as we did in the Competition and Innovation" lecture. As all firms face the same problem, solve for a generic firm's solution - once you take the derivative with respect to the firm's effort level, invoke symmetry that x1 = x2 = x'. The resulting x* will characterize the behavior of all firms in the race.) b. Solve for the optimal level of effort if n=4. c. How does the optimal level of effort exerted by each firm change as more firms effort? What does this tell us about the role of competition in incentivizing innovation