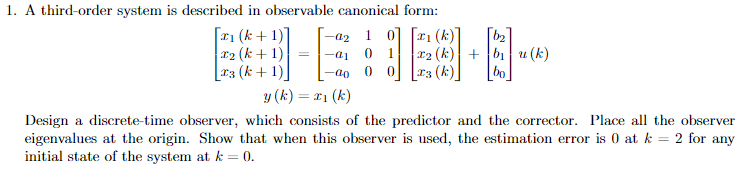
1. A third-order system is described in observable canonical form: [x1 (k+1)] -a2 1 0] [1(k) x2 (k+1) x3 (k+ = -a1 0 1 -ao 0 0 (k) bu(k) 23(k) y (k) = x1 (k) Design a discrete-time observer, which consists of the predictor and the corrector. Place all the observer eigenvalues at the origin. Show that when this observer is used, the estimation error is 0 at k = 2 for any initial state of the system at k = 0.