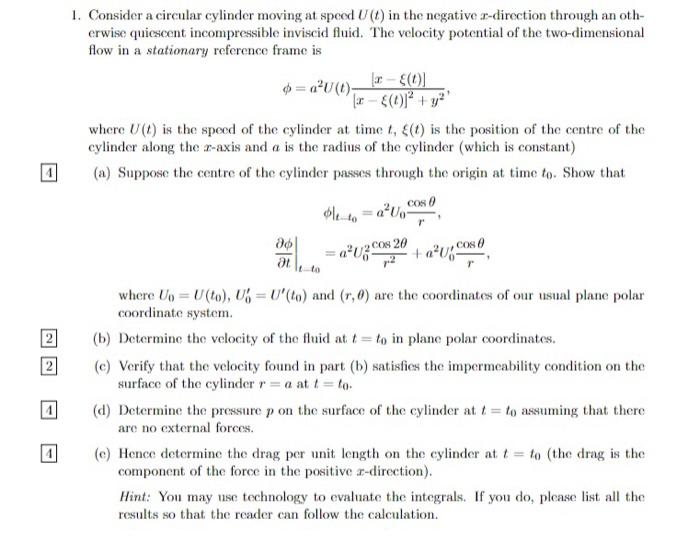
1. Consider a circular cylinder moving at speed U(t) in the negative z-direction through an oth- erwise quiescent incompressible inviscid fluid. The velocity potential of the two-dimensional flow in a stationary reference frame is $=aU(t)- [r-{(t)] r (1)]+ y where U(t) is the speed of the cylinder at time t, (t) is the position of the centre of the cylinder along the z-axis and a is the radius of the cylinder (which is constant) (a) Suppose the centre of the cylinder passes through the origin at time to. Show that cos 0 r ole-to 3acos 20+ au.co = at where Up = U(to), U = U'(to) and (r, 0) are the coordinates of our usual plane polar coordinate system. (b) Determine the velocity of the fluid at t= to in plane polar coordinates. (c) Verify that the velocity found in part (b) satisfies the impermeability condition on the surface of the cylinder r= a at t = to. (d) Determine the pressure p on the surface of the cylinder at t= to assuming that there are no external forces. (e) Hence determine the drag per unit length on the cylinder at t= to (the drag is the component of the force in the positive z-direction). Hint: You may use technology to evaluate the integrals. If you do, please list all the results so that the reader can follow the calculation.