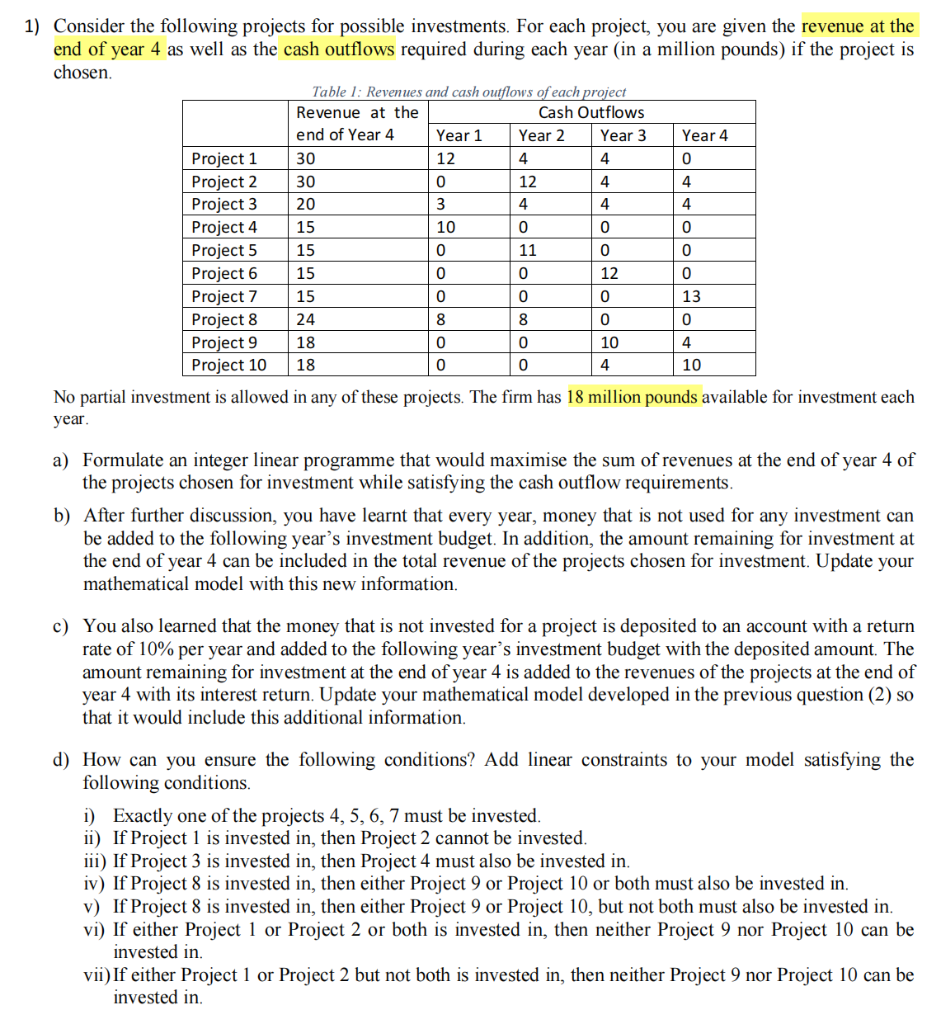
1) Consider the following projects for possible investments. For each project, you are given the revenue at the end of year 4 as well as the cash outflows required during each year (in a million pounds) if the project is chosen. No partial investment is allowed in any of these projects. The firm has 18 million pounds available for investment each year. a) Formulate an integer linear programme that would maximise the sum of revenues at the end of year 4 of the projects chosen for investment while satisfying the cash outflow requirements. b) After further discussion, you have learnt that every year, money that is not used for any investment can be added to the following year's investment budget. In addition, the amount remaining for investment at the end of year 4 can be included in the total revenue of the projects chosen for investment. Update your mathematical model with this new information. c) You also learned that the money that is not invested for a project is deposited to an account with a return rate of 10% per year and added to the following year's investment budget with the deposited amount. The amount remaining for investment at the end of year 4 is added to the revenues of the projects at the end of year 4 with its interest return. Update your mathematical model developed in the previous question (2) so that it would include this additional information. d) How can you ensure the following conditions? Add linear constraints to your model satisfying the following conditions. i) Exactly one of the projects 4, 5, 6, 7 must be invested. ii) If Project 1 is invested in, then Project 2 cannot be invested. iii) If Project 3 is invested in, then Project 4 must also be invested in. iv) If Project 8 is invested in, then either Project 9 or Project 10 or both must also be invested in. v) If Project 8 is invested in, then either Project 9 or Project 10 , but not both must also be invested in. vi) If either Project 1 or Project 2 or both is invested in, then neither Project 9 nor Project 10 can be invested in. vii) If either Project 1 or Project 2 but not both is invested in, then neither Project 9 nor Project 10 can be 1) Consider the following projects for possible investments. For each project, you are given the revenue at the end of year 4 as well as the cash outflows required during each year (in a million pounds) if the project is chosen. No partial investment is allowed in any of these projects. The firm has 18 million pounds available for investment each year. a) Formulate an integer linear programme that would maximise the sum of revenues at the end of year 4 of the projects chosen for investment while satisfying the cash outflow requirements. b) After further discussion, you have learnt that every year, money that is not used for any investment can be added to the following year's investment budget. In addition, the amount remaining for investment at the end of year 4 can be included in the total revenue of the projects chosen for investment. Update your mathematical model with this new information. c) You also learned that the money that is not invested for a project is deposited to an account with a return rate of 10% per year and added to the following year's investment budget with the deposited amount. The amount remaining for investment at the end of year 4 is added to the revenues of the projects at the end of year 4 with its interest return. Update your mathematical model developed in the previous question (2) so that it would include this additional information. d) How can you ensure the following conditions? Add linear constraints to your model satisfying the following conditions. i) Exactly one of the projects 4, 5, 6, 7 must be invested. ii) If Project 1 is invested in, then Project 2 cannot be invested. iii) If Project 3 is invested in, then Project 4 must also be invested in. iv) If Project 8 is invested in, then either Project 9 or Project 10 or both must also be invested in. v) If Project 8 is invested in, then either Project 9 or Project 10 , but not both must also be invested in. vi) If either Project 1 or Project 2 or both is invested in, then neither Project 9 nor Project 10 can be invested in. vii) If either Project 1 or Project 2 but not both is invested in, then neither Project 9 nor Project 10 can be







