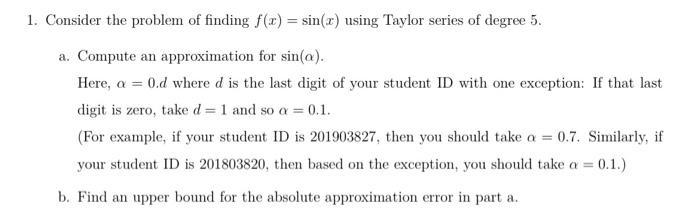
1. Consider the problem of finding f(x) = sin(x) using Taylor series of degree 5. a. Compute an approximation for sin(a). Here, a = 0.d where d is the last digit of your student ID with one exception: If that last digit is zero, take d = 1 and so a = 0.1. (For example, if your student ID is 201903827, then you should take a = 0.7. Similarly, if your student ID is 201803820, then based on the exception, you should take a = t = 0.1.) b. Find an upper bound for the absolute approximation error in part a.