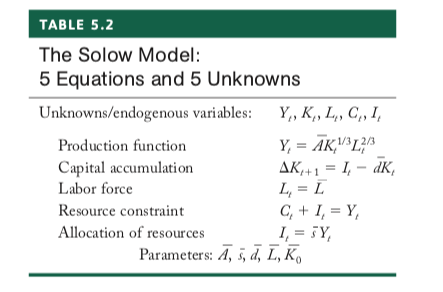
1. Consider the Solow model. Let the resource constraint be given by Y = C + I, where Y = AK, L is production at time t, Of is consumption, I, is investment, K, is capital, L, is labor, and A is productivity. Let the capital accumulation equation be given by Kit1 - K = ], -dK,, where d is the rate of capital depreciation. Suppose that investment is equal to 1 = 3), where s is the percentage of output that is invested. Suppose that labor is exogenous and equal to _ (that is, L = _ in every period). (a) Let the steady state value for capital be denoted by K*. Solve for the steady state value of capital as a function of the exogenous parameters. Show every step of all calculations. (b) Let the steady state value for output be denoted by Y"*. Solve for the steady state value of output as a function of the exogenous parameters. Show every step of all calculations. (c) Solve for the steady state value of the capital-output ratio, , as a function of the exogenous parameters. Show every step of all calculations. (d) Use your answer to part c) to compute the steady state value of the capital-output ratio when 8 = 0.25 and d = 0.1. Similarly, compute the steady state value of the capital-output ratio when 8 = 0.35 and d = 0.05. (e) Draw the Solow diagram. That is, make a graph where you plot investment, depreciation, and output (with the capital stock on the horizontal axis). Highlight the steady state value for capital in the graph. Show graphically what the value for consumption is when capital is equal to its steady state value. (f) Now draw a new Solow diagram where you show what happens to output and capital if produc- tivity, A, permanently declines. Comment on the results. (g) Does the growth rate of output stay constant as output converges from the original steady state to the new steady state in part f) or does the growth rate of output vary over time? Comment on the result.TABLE 5.2 The Solow Model: 5 Equations and 5 Unknowns Unknowns/endogenous variables: Y, K, L, C,, I, Production function Y, = AK,13LY Capital accumulation AK,+1 = I, - dK Labor force L = L Resource constraint C+I =Y, Allocation of resources I, = FY, Parameters: A, 5, d, L, Ko