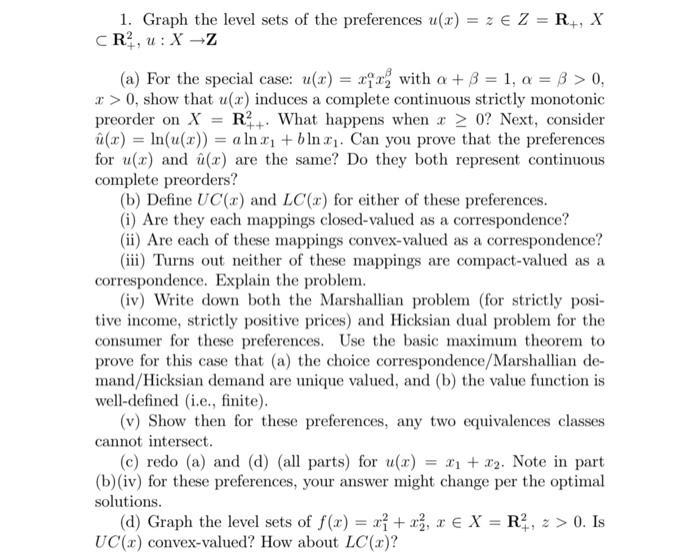
1. Graph the level sets of the preferences u(x) = z Z = R+, X CR, u: X-Z (a) For the special case: u(x) = 2 with a + 3 = 1, a = 3 > 0, x> 0, show that u(x) induces a complete continuous strictly monotonic preorder on X = R2. What happens when a 20? Next, consider (x) = ln(u(x)) = alnx + bln x. Can you prove that the preferences for u(x) and u(x) are the same? Do they both represent continuous complete preorders? (b) Define UC(x) and LC(x) for either of these preferences. (i) Are they each mappings closed-valued as a correspondence? (ii) Are each of these mappings convex-valued as a correspondence? (iii) Turns out neither of these mappings are compact-valued as a correspondence. Explain the problem. (iv) Write down both the Marshallian problem (for strictly posi- tive income, strictly positive prices) and Hicksian dual problem for the consumer for these preferences. Use the basic maximum theorem to prove for this case that (a) the choice correspondence/Marshallian de- mand/Hicksian demand are unique valued, and (b) the value function is well-defined (i.e., finite). (v) Show then for these preferences, any two equivalences classes cannot intersect. (c) redo (a) and (d) (all parts) for u(x) = x1 + x2. Note in part (b)(iv) for these preferences, your answer might change per the optimal solutions. (d) Graph the level sets of f(x) = x + x2, x = X = R, z> 0. Is UC(a) convex-valued? How about LC(x)?