Question
1. (i.) Justify the following statement: the total degree (sum of all degrees of all vertices) of a finite graph is an isomorphism invariant.
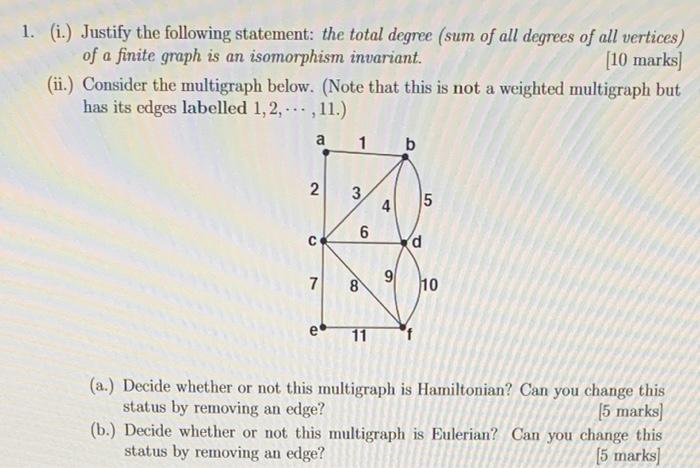
1. (i.) Justify the following statement: the total degree (sum of all degrees of all vertices) of a finite graph is an isomorphism invariant. [10 marks] (ii.) Consider the multigraph below. (Note that this is not a weighted multigraph but has its edges labelled 1,2,..., 11.) a 1 b 2 3 4 6 0 7 8 s d 9 10 e 11 (a.) Decide whether or not this multigraph is Hamiltonian? Can you change this status by removing an edge? [5 marks] (b.) Decide whether or not this multigraph is Eulerian? Can you change this status by removing an edge? [5 marks]
Step by Step Solution
There are 3 Steps involved in it
Step: 1

Get Instant Access to Expert-Tailored Solutions
See step-by-step solutions with expert insights and AI powered tools for academic success
Step: 2

Step: 3

Ace Your Homework with AI
Get the answers you need in no time with our AI-driven, step-by-step assistance
Get StartedRecommended Textbook for
International Financial Reporting and Analysis
Authors: David Alexander, Anne Britton, Ann Jorissen
5th edition
978-1408032282, 1408032287, 978-1408075012
Students also viewed these Mathematics questions
Question
Answered: 1 week ago
Question
Answered: 1 week ago
Question
Answered: 1 week ago
Question
Answered: 1 week ago
Question
Answered: 1 week ago
Question
Answered: 1 week ago
Question
Answered: 1 week ago
Question
Answered: 1 week ago
Question
Answered: 1 week ago
Question
Answered: 1 week ago
Question
Answered: 1 week ago
Question
Answered: 1 week ago
Question
Answered: 1 week ago
Question
Answered: 1 week ago
Question
Answered: 1 week ago
Question
Answered: 1 week ago
Question
Answered: 1 week ago
Question
Answered: 1 week ago
Question
Answered: 1 week ago
Question
Answered: 1 week ago
Question
Answered: 1 week ago
Question
Answered: 1 week ago
Question
Answered: 1 week ago
View Answer in SolutionInn App



