Question: 1. If a is a real constant, determine the volume V of the parallelepiped spanned by the vectors (a, -3,0), (5, a, 2), (1,5,

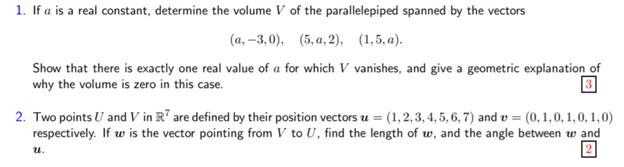
1. If a is a real constant, determine the volume V of

1. If a is a real constant, determine the volume V of the parallelepiped spanned by the vectors (a, -3,0), (5, a, 2), (1,5, a). Show that there is exactly one real value of a for which V vanishes, and give a geometric explanation of why the volume is zero in this case. 2. Two points U and V in R' are defined by their position vectors u = (1,2,3, 4, 5,6, 7) and v = (0,1,0, 1,0, 1,0) respectively. If w is the vector pointing from V to U, find the length of w, and the angle between w and u.

Step by Step Solution

★★★★★

3.43 Rating (150 Votes )

There are 3 Steps involved in it

1 Expert Approved Answer

Step: 1 Unlock

Lets solve the problems step by step Problem 1 To determine the volume V of the parallelepiped spann... View full answer

Question Has Been Solved by an Expert!

Get step-by-step solutions from verified subject matter experts

Step: 2 Unlock

Step: 3 Unlock

Students Have Also Explored These Related Mathematics Questions!

Activity 1: Newton's Second Law Data Table 1 Suspended Weight of Time Average Average d (m) 2d. Time2 Mass (kg) Suspended (sec) Time Mass (mass x 9.8 m/s), Newtons 3 Washers Trial 1: Trial 2: Trial...

Give a geometric explanation of why a homogeneous linear system consisting of two equations in three unknowns must have infinitely many solutions. What are the possible numbers of solutions for a...

The volume V of a given mass of a gas varies directly as the temperature T and inversely as the pressure P. If V = 231 cm3 when T = 42o and P = 20 kg/cm2, what is the volume when T = 30o and P = 15...

The volume V of liquid in a spherical tank of radius r is related to the depth h of the liquid by V = h2(3r h)/3 Determine h given r = 1 m and V = 0.75 m3.

Name : Symara B Section: Date: Archimedes' Principle Objectives 1) To verify Archimedes' Principle by comparing the buoyant force on a submerged object and the weight of the fluid that it displaces....

Portray in words what transforms you would have to make to your execution to some degree (a) to accomplish this and remark on the benefits and detriments of this thought.You are approached to compose...

answer the question clearly You are building a flight-control system for which a convincing safety case must be made. Would you assign the tasks of safety requirements engineering, test case...

Calculus 3 Sections 12.1 and 12.2 Reading Assignment: 3D Coordinates and Vectors Answer Only Exercise 1 by using a screenshot provided Calculus Pearson textbook. Make sure you read these three...

space weather PLEASE HELP ME use MATLAB NO REFERENCE 1.1 Free Space Maxwell's equations describe the behavior of electromagnetic fields and their interaction with mat- ter. They are one of the major...

QUIZ... Let D be a poset and let f : D D be a monotone function. (i) Give the definition of the least pre-fixed point, fix (f), of f. Show that fix (f) is a fixed point of f. [5 marks] (ii) Show that...

1 Dimensions and Units 1.1 Physical Dimensions and Units of Measurement You've probably heard the expression that you can't compare apples and oranges. To a physicist, the key concept underlying this...

The following information was extracted from the accounting records of Pickin and Grinnin, LLC, a manufacturer of guitars: Beginning Raw Materials Inventory $375,000 Ending Raw Materials Inventory...

The current gain for a transistor working as common-base amplifier is 0.96. If the emitter current is 7.2 mA, then the base current is [1996] (a) 0.29 mA (c) 0.39 mA (b) 0.35 mA (d) 0.43 mA

8. Consider a sampled-data system with the closed-loop system transfer function z +2z T(z) = K z + 0.2z-0.5 This system is: a. Stable for all finite K. b. Stable for -0.5

In evaluating the performance ( returns ) of a U . S . Large Cap Stock Mutual Fund, the index / benchmark that would be least appropriate for comparisons sake would be _ _ _ _ _ . Group of answer...

Problem 10-7A (Algo) Natural resources LO P3 [The following information applies to the questions displayed below.] On July 23 of the current year, Dakota Mining Company pays $7,852,800 for land...

Using a Residual Income Model (RIM), where residual income grows at rate 8 for n=6 years and rate 8 thereafter, compute the intrinsic value per share for Acme Inc. given the following information...

PLEASE SOLVE IN EXCEL WITH FORMULAS!! A truck must travel from New York to Los Angeles. As shown in the diagram, several routes are available. The number associated with each arc is the number of...

Determine the theoretical and actual material required to produce the part, shown in figure. Raw stock is supplied in 0.875 in. diameter. A lathe cutoff tool width is 0 125 in. A 0.015 in. stock...

In the case, "Mobil Oil Exploration & Producing Southeast, Inc. v. United States, 530 U.S. 604 (2000)." Identify the specific areas of business law relevant to contract law.

The problem for finding a largest block is described in Programming Exercise 8.35. Design a dynamic programming algorithm for solving this problem in O(n2) time. Write a test program that displays a...

9.1 Exercise (JDK Source Code): Extract the source code of the class Math from the JDK source code ("$JAVA_HOME" "src.zip" "Math.java" under folder "java.lang"). Study how constants such as E and...

To better see how the growth rate evolves through time, plot the annualized growth rate of consumption expenditure. Describe what you see. Is it constant on average? Please use this data: Year...

My code is encountering an error: Traceback (most recent call last): File "main.py", line 18, in age = int(record[1]) IndexError: string index out of range The code an assignment are below: file=...

Eli Orchid has designed a new pharmaceutical product, Orchid Relief, which improves the night sleep. Before initiating mass production of the product, Eli Orchid has been market-testing Orchid Relief...

For example, if printSparseTable(4, 10); is called, you would print: 4 5 5 7 6 8 8 10 9 13 Note that even though fibby(3) == fibby(4), since we didn't print fibby(3), we still print fibby(4). But we...