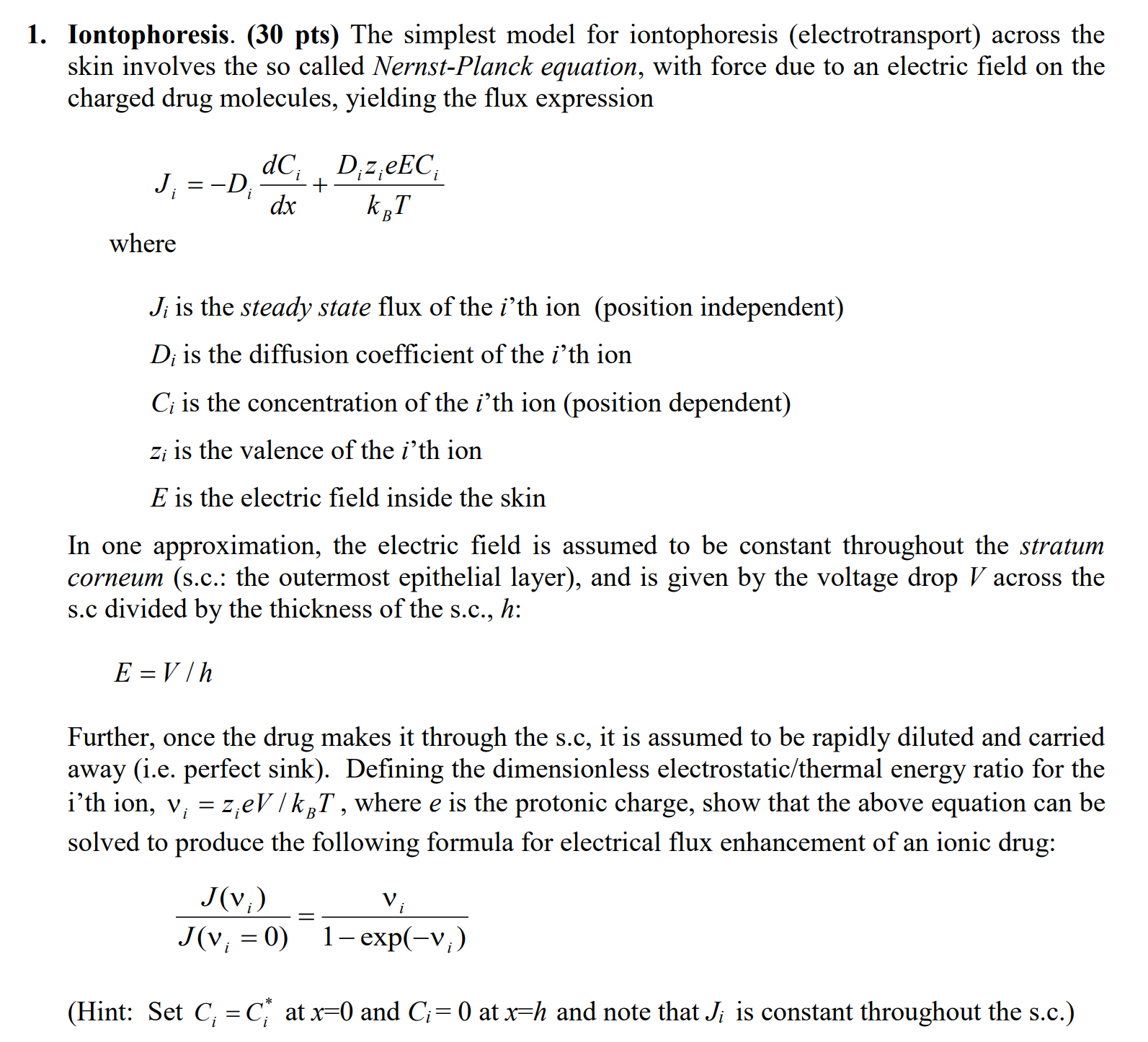
1. Iontophoresis. (30 pts) The simplest model for iontophoresis (electrotransport) across the skin involves the so called Nernst-Planck equation, with force due to an electric field on the charged drug molecules, yielding the flux expression i J = D; dC, dx D,z,eEC + B kT where J; is the steady state flux of the i'th ion (position independent) D; is the diffusion coefficient of the i'th ion C; is the concentration of the i'th ion (position dependent) Zi is the valence of the i'th ion E is the electric field inside the skin In one approximation, the electric field is assumed to be constant throughout the stratum corneum (s.c.: the outermost epithelial layer), and is given by the voltage drop V across the s.c divided by the thickness of the s.c., h: E=V/h Further, once the drug makes it through the s.c, it is assumed to be rapidly diluted and carried away (i.e. perfect sink). Defining the dimensionless electrostatic/thermal energy ratio for the i'th ion, v; = z;eV / kT, where e is the protonic charge, show that the above equation can be solved to produce the following formula for electrical flux enhancement of an ionic drug: J(v,) J(v; = 0) Vi 1-exp(v;) (Hint: Set C = C* at x=0 and C = 0 at x=h and note that J; is constant throughout the s.c.)