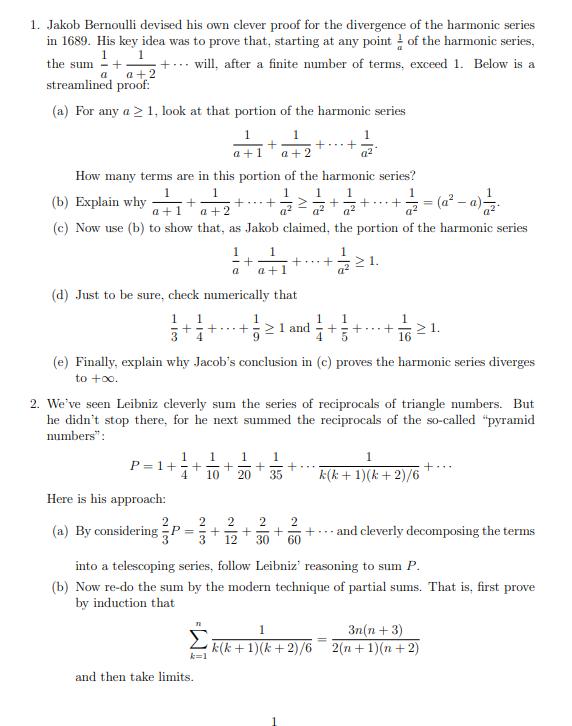
1. Jakob Bernoulli devised his own clever proof for the divergence of the harmonic series in 1689. His key idea was to prove that, starting at any point of the harmonic series, the sum + +... will, after a finite number of terms, exceed 1. Below is a 1 1 a a+2 streamlined proof: (a) For any a 1, look at that portion of the harmonic series 1 1 + a+1 a+2 How many terms are in this portion of the harmonic series? 1 (b) Explain why 1+2+ ++ 1 1 1 aa +1/12 a (c) Now use (b) to show that, as Jakob claimed, the portion of the harmonic series ++ 1/1/ 1 + a a+1 (d) Just to be sure, check numerically that 1 1 3+1 1 1 +.. + 4+3 1. (e) Finally, explain why Jacob's conclusion in (c) proves the harmonic series diverges to +o. Here is his approach: (a) By considering P = 1 1 1 1 1 P=1+=+ + + + 4 10 20 35 213 2. We've seen Leibniz cleverly sum the series of reciprocals of triangle numbers. But he didn't stop there, for he next summed the reciprocals of the so-called "pyramid numbers": ++ +/ n and then take limits. 21 and k=1 + + 2 2 2 + + + 12 30 60 + a ++ 1 k(k+1)(k+2)/6 into a telescoping series, follow Leibniz' reasoning to sum P. (b) Now re-do the sum by the modern technique of partial sums. That is, first prove by induction that 1 k(k+ 1)(k+2)/6 +... and cleverly decomposing the terms +. 3n(n+3) 2(n + 1)(n+2)