Question
1. Let C denote the field of complex numbers. As with any field, we can consider vector spaces, linear transformations, and matrices over C rather
1. Let C denote the field of complex numbers. As with any field, we can consider vector spaces, linear transformations, and matrices over C rather than over our usual field R. (a) The complex numbers C can be viewed as a vector space over either C or R with the usual scalar multiplication. Prove that C has dimension 1 as a vector space over C but has dimension 2 as a vector space over R. In each case, give an explicit basis.
(b) Diagonalize the following matrices over C by giving QA, QB ? M22(C) so that Q ?1 A AQA and Q ?1 B BQ are diagonal. A = 0 1 ?1 0 , B = 1 1 ?1 0
7. Suppose that A ? Mnn(R) has two distinct eigenvalues ?1 and ?2 with dim(E?1 ) = n ? 1. Prove that A is diagonalizable.
And Part b and d in jpg. plz show detailed and clearly works. Make sure correct!! thanks
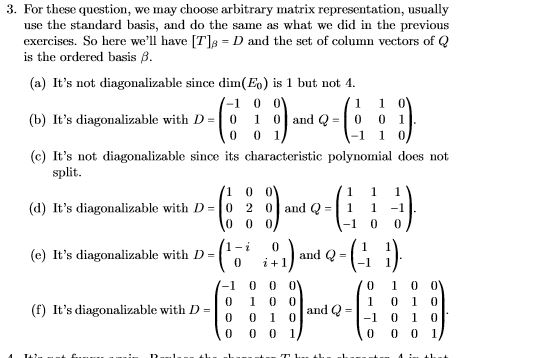
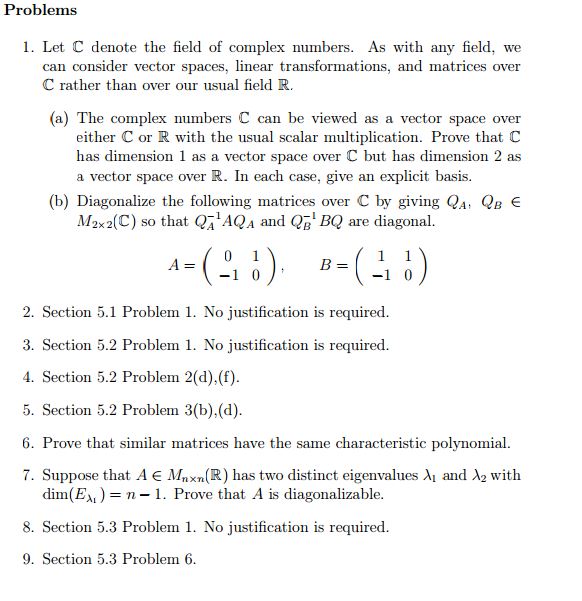
Step by Step Solution
There are 3 Steps involved in it
Step: 1

Get Instant Access to Expert-Tailored Solutions
See step-by-step solutions with expert insights and AI powered tools for academic success
Step: 2

Step: 3

Ace Your Homework with AI
Get the answers you need in no time with our AI-driven, step-by-step assistance
Get Started


