1) (made-up data)Confidence Interval Problem for Proportions: Calculate a 95% confidence interval for these proportions. SHOW YOUR "E" SETUP & CALCULATIONS. The same assumptions must be met to ensure the statistical analysis is valid and Make sure to calculate the proportions first.

High school students take the AP tests in different subject areas. This year 150 students took the biology exam of which 85 were female. And, 220 students took the calculus exam of which 100 were female. Calculate the difference in the PROPORTION of female students taking the biology exam versus female students taking the calculusexam using a 95% confidence level.

E = z-critical * sqrt[ (p-hat1 * q-hat1)1) + (p-hat2 * q-hat2)2)]

2) (made-up data)Hypothesis Test problem for Proportions: Assume a 1% significance level. The same assumptions must be met to ensure the statistical analysis is valid and calculate the proportions first.

SHOW Z-Test SETUP AND Z-Critical value the z-Test was compared to. Also, use software to calculate the p-Value. Do both support the same conclusion? (they MUST). State your real-world conclusion?

Are more children diagnosed with Autism Spectrum Disorder in States that have urban areas over States that are mostly rural? In an urban State, there were are 300 3^rd grade students diagnosed with ASD out of 18,500 3^rd graders tested. In a rural state, there were 55 3^rd grade students diagnosed with ASD out of 2,300 3^rd graders tested. Is there enough evidence to show that the proportion of children diagnosed with ASD in an urban State is more than the proportion in a rural State? Test at the = 1% level.

4) (made-up data) Hypothesis Test problem for TWO population DIFFERENCES (not proportions or means) comparison hypothesis test (NOT a proportion problem - so we use t-values, not z-values). SHOW t-TEST SETUP and calculation, AND for the t-Critical, what SIGNIFICANCE LEVEL (the column) and what df (which row) were used and what the t-Critical value is.

Do people avoid driving on Friday the 13^th? Here are data on 20 Fridays: 10 on the 13^th and the other 10 on the Friday before. For the DIFFERENCES, Calculate the MEAN and SD between traffic on these two Fridays at the 95% level(alpha = 5%);

Traffic Count

Dates	6th (x1)	13th (x2)	d = (x1 - x2)
1990, July	139246	138548
1990, July	138012	132908
1991, September	137055	136018
1991, September	140675	131843
1991, December	123552	121641
1991, December	121139	120723
1992, March	128293	125532
1992, March	124631	120249
1992, November	124609	125770
1992, November	119584	116263

For the differences (all d's) the MEAN = _______ and the SD = __________

TEST STATISTIC: t-Test = (d-bar -_d)/s_d /sqrt(n) = ____; The t-Critical with (n-1) df for = 5% is______

What is the software generated p-value for the t-Test? ______

What is your math conclusion and your real-world conclusion?

5) Confidence Interval problem for two population DIFFERENCES (not means or proportions) that have the SAME NUMBER OF DATA VALUES (n1 = n2). Using the same traffic count data and statistics as above, the margin of error, E, formula is E = t-critical * s_d/sqrt(n) We will assume a significance level of = 5%(95% confidence level) with n=10 data points, hence df = (10 - 1 ) = 9, so t-critical =______ ? What are the MEAN ______and SD _____for the "differences"?

E = _______ * _______ / ______ = __________ (fill in the different values and the calculated result) .

Our Confidence Intervalis (d-bar - E) d d

Real-world Conclusion: Whose median income mean is larger and by how much?

(IF this or any CI includes zero there is NO difference)

(5) (made-up data) Hypothesis Test problem comparing two population MEANS. Make sure to show your setup for the t-Test, df and how you found the t-Critical.Also, use software to find the p-value (probability) is it larger or smaller than ? Does this conclusion match the one based on the t-Test verses t-Critical? (It must). For the p-value, make sure you know which area (right or left) you need to use (greater than or less than). (If NO Confidence Level or is provided assume 95% and 5%, respectively)

Median Income for Males

$42,951	$52,379	$42,544	$37,488	$49,281	$50,987	$60,705
$50,411	$66,760	$40,951	$43,902	$45,494	$41,528	$50,746
$45,183	$43,624	$43,993	$41,612	$46,313	$43,944	$56,708
$60,264	$50,053	$50,580	$40,202	$43,146	$41,635	$42,182
$41,803	$53,033	$60,568	$41,037	$50,388	$41,950	$44,660

Median Income for Females

$31,862	$40,550	$36,048	$30,752	$41,817	$40,236	$47,476
$60,332	$33,823	$35,438	$37,242	$31,238	$39,150	$34,023
$33,269	$32,684	$31,844	$34,599	$48,748	$46,185	$36,931
$29,548	$33,865	$31,067	$33,424	$35,484	$41,021	$47,155
$42,113	$33,459	$32,462	$35,746	$31,274	$36,027	$37,089

t-Test = [(x-bar1 - x-bar2) -(1 - 2)] / sqrt ( s₁²1 + s₂²2)

To find the t-Critical we need the degrees of freedom (df) and the formula to calculate df is "hairy". As presented earlier, there simpler formula that is used by some statisticians: df = (n1 - 1) + (n2 - 1)

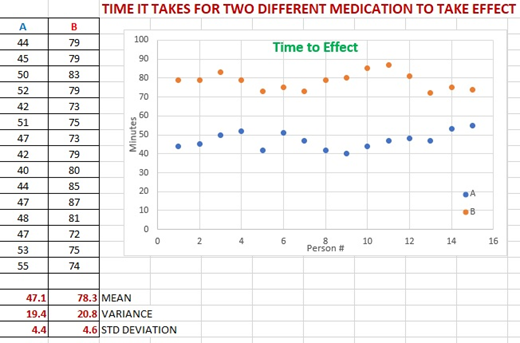
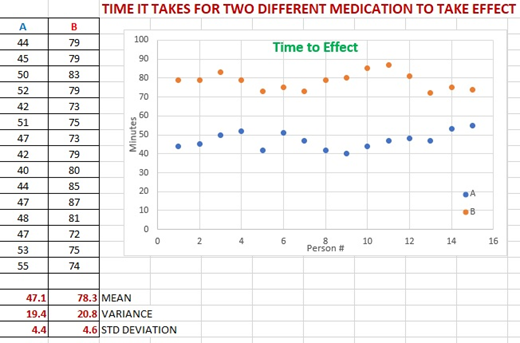
6) AnotherHypothesis Test problem comparing two population MEANS for the two-medication "time to take effect" example. Work it just as you did the prior problem (#5)

t-Test = [(x-bar1 - x-bar2) -(1 - 2)] / sqrt ( s₁²1 + s₂²2)

To find the t-Critical we need the degrees of freedom (df): df = (n1 - 1) + (n2 - 1)

Also, find the p-value

Bottom line: Is there a significant difference between the mean "time to take effect" for these two medications and if so, which medication is significantly faster.

TIME IT TAKES FOR TWO DIFFERENT MEDICATION TO TAKE EFFECT A B 44 79 100 Time to Effect 45 79 90 50 83 80 52 79 70 42 73 60 51 75 Minutes 47 73 150 42 79 40 40 80 30 44 85 20 47 87 10 48 81 47 72 2 4 6 10 12 14 16 53 75 Person # 55 74 47.1 78.3 MEAN 19.4 20.8 VARIANCE 4.4 4.6 STD DEVIATION