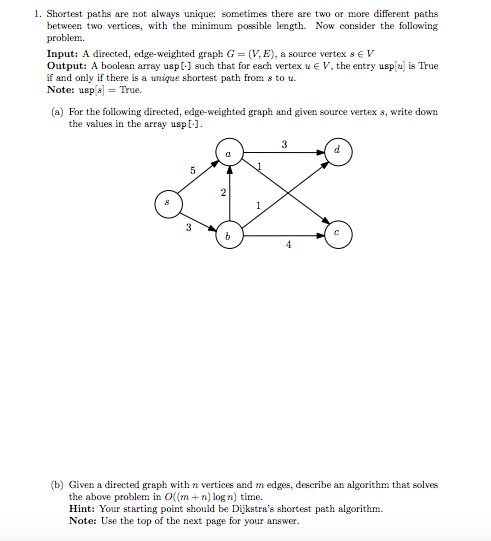
1. Shortest paths are not always unique: sometimes there are two or more different paths between two vertices, with the minimum possible length. Now consider the following problem Input: A directed, edge-weighted graph G = (V. E), a source vertex s E V Output: A boolean array usp[-] such that for each vertex u E V, the entry usp[u is True if and only if there is a unique shortest path from s to . Note: usp sTrue. (a) For the following directed, edge-weighted graph and given source vertex s, write down the values in the array uspt- (b) Given a directed graph with n vertices and m edges, describe an algorithm that solves the above proble in O(m) log n) time. Hint: Your starting point should be Dijkstra's shortest path algorthm Note: Use the top of the next page for your