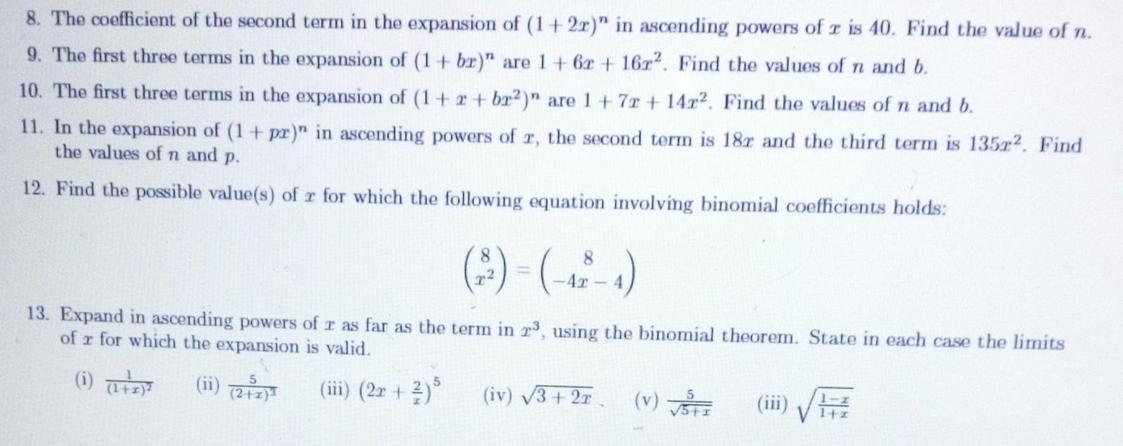
1. Simplify (3) + (6) + (2) giving your answer as a single binomial coefficient of the form 2. Find the expansion of (i) (3r-4y)4 (x - 1)8 3. Expand (a + b)5. If a = 3 and b = 1, find the value (as a fraction) of the fourth term of the expansion. (i) Write down the expansion of (2 + x) and (2-r). 4. (ii) Hence, simplify (2+2)4+ (2-r) 4. Use your results to find the exact value of (2+3)4+ (2-3)4 5. Determine the value of each of the following correct to five significant figures using the binomial theorem. (i) (0.98) (ii) (1.003)5 (iii) (2.01)9 6. (i) Determine the sixth term in the expansion of (32+)3 13 (ii) Determine the middle term of (2r - 52)8 (ii) Determine the term independent of r in the expansion of (iv) Determine the term containing 2-12 in the expansion of of (22-)20 277)5 7. Evaluate the coefficients of r and 25 in the expansion of (-3). Hence, evaluate the coefficient of 25 in the expansion of (-3) (r +6) 8. The coefficient of the second term in the expansion of (1+2r)" in ascending powers of r is 40. Find the value of n. 9. The first three terms in the expansion of (1 + br)" are 1 +62 + 16r. Find the values of n and b. 10. The first three terms in the expansion of (1+2+ br2)n are 1 +7r+14r. Find the values of n and b. 11. In the expansion of (1 + pr)" in ascending powers of r, the second term is 18r and the third term is 135r2. Find the values of n and p. 12. Find the possible value(s) of r for which the following equation involving binomial coefficients holds: 13. Expand in ascending powers of r as far as the term in 23, using the binomial theorem. State in each case the limits of r for which the expansion is valid. (i) (a+z) 2 (ii) (2) (iii) (2x +)5 (iv) 3+2r (V) VSTI (iii)17 V 1+z