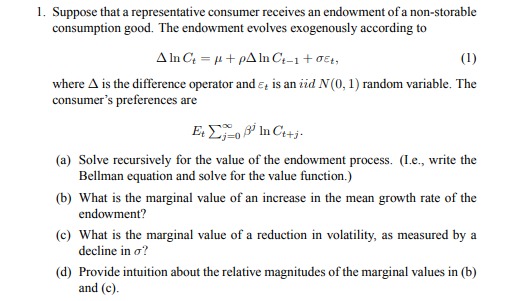
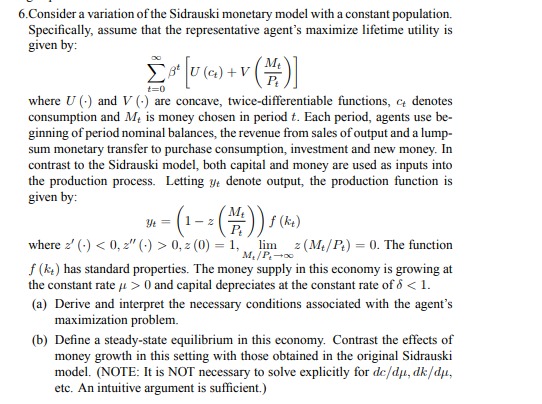
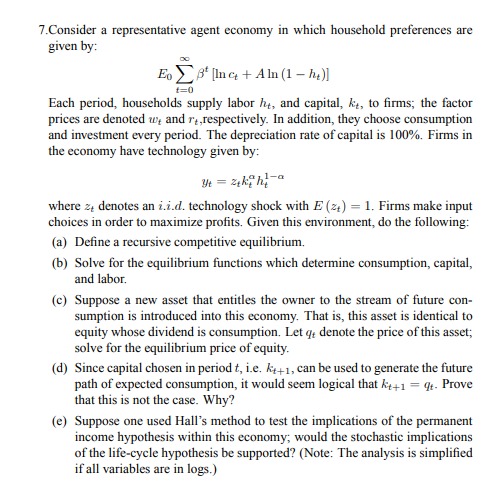
1. Suppose that a representative consumer receives an endowment of a non-storable consumption good. The endowment evolves exogenously according to Aln Ce = p + pAlnot-1+ 0Et, (1) where A is the difference operator and e, is an rid NV(0, 1) random variable. The consumer's preferences are Et Cj=of' In Ctti. (a) Solve recursively for the value of the endowment process. (Le., write the Bellman equation and solve for the value function.) (b) What is the marginal value of an increase in the mean growth rate of the endowment? (c) What is the marginal value of a reduction in volatility, as measured by a decline in o? (d) Provide intuition about the relative magnitudes of the marginal values in (b) and (c).6. Consider a variation of the Sidrauski monetary model with a constant population. Specifically, assume that the representative agent's maximize lifetime utility is given by: [ At U ( c) + V ()] 1=0 where U () and V () are concave, twice-differentiable functions, a denotes consumption and M, is money chosen in period t. Each period, agents use be- ginning of period nominal balances, the revenue from sales of output and a lump- sum monetary transfer to purchase consumption, investment and new money. In contrast to the Sidrauski model, both capital and money are used as inputs into the production process. Letting y denote output, the production function is given by: = f ( kit ) where z' (.) 0,= (0) =1, lim =(M/P) = 0. The function "M. /P. -+30 f (ke ) has standard properties. The money supply in this economy is growing at the constant rate / > 0 and capital depreciates at the constant rate of 6 B' [Ince + Alm (1 - he)] 1 = 0 Each period, households supply labor he, and capital, ke, to firms; the factor prices are denoted w, and re,respectively. In addition, they choose consumption and investment every period. The depreciation rate of capital is 100%. Firms in the economy have technology given by: where z denotes an i.i.d. technology shock with E (2,) = 1. Firms make input choices in order to maximize profits. Given this environment, do the following: (a) Define a recursive competitive equilibrium. (b) Solve for the equilibrium functions which determine consumption, capital, and labor. (c) Suppose a new asset that entitles the owner to the stream of future con- sumption is introduced into this economy. That is, this asset is identical to equity whose dividend is consumption. Let q, denote the price of this asset; solve for the equilibrium price of equity. (d) Since capital chosen in period t, i.e. At+1, can be used to generate the future path of expected consumption, it would seem logical that ke+1 = qf. Prove that this is not the case. Why? (e) Suppose one used Hall's method to test the implications of the permanent income hypothesis within this economy; would the stochastic implications of the life-cycle hypothesis be supported? (Note: The analysis is simplified if all variables are in logs.)












