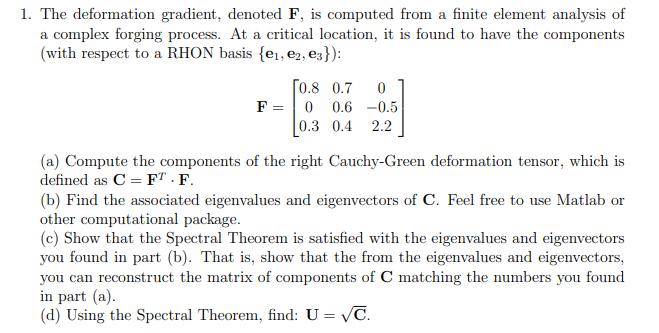
1. The deformation gradient, denoted F, is computed from a finite element analysis of a complex forging process. At a critical location, it is found to have the components (with respect to a RHON basis (e1, e2, e3}): [0.8 0.7 F= 0 0 0.6-0.5 0.3 0.4 2.2 (a) Compute the components of the right Cauchy-Green deformation tensor, which is defined as CFT.F. (b) Find the associated eigenvalues and eigenvectors of C. Feel free to use Matlab or other computational package. (c) Show that the Spectral Theorem is satisfied with the eigenvalues and eigenvectors you found in part (b). That is, show that the from the eigenvalues and eigenvectors, you can reconstruct the matrix of components of C matching the numbers you found in part (a). (d) Using the Spectral Theorem, find: U = C.