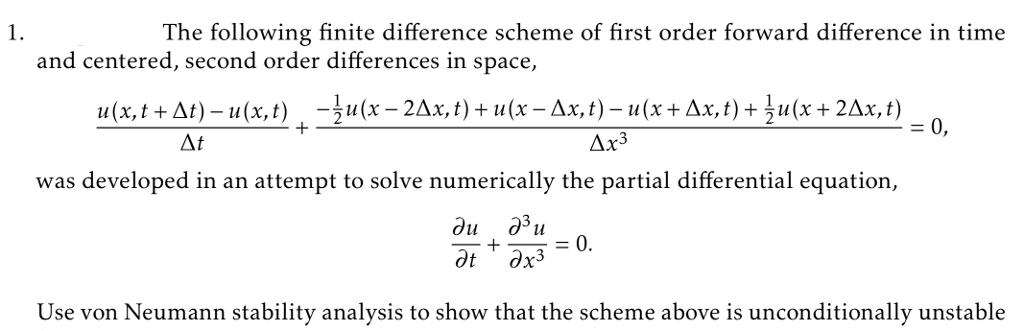
1. The following finite difference scheme of first order forward difference in time and centered, second order differences in space, u(x,t + At) - u(x, t) -u(x-2Ax, t) + u(x-Ax, t) - u(x+Ax, t) + u(x+2^x, t) + = 0, At Ax was developed in an attempt to solve numerically the partial differential equation, 2 u x3 Use von Neumann stability analysis to show that the scheme above is unconditionally unstable at + = 0.