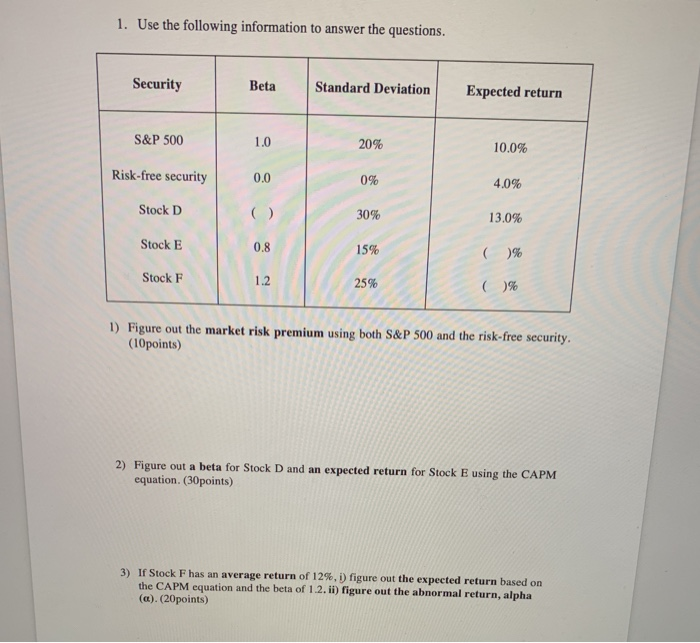
1. Use the following information to answer the questions. Security Beta Standard Deviation Expected return S&P 500 20% 10.0% Risk-free security 0% 4.0% Stock D 30% 13.0% Stock E 15% ( )% Stock F 25% ( )% 1) Figure out the market risk premium using both S&P 500 and the risk-free security. (10points) 2) Figure out a beta for Stock D and an expected return for Stock E using the CAPM equation. (30points) 3) I Stock F has an average return of 12%, i) figure out the expected return based on the CAPM equation and the beta of 1.2. ii) figure out the abnormal return, alpha (a). (20points) 4) You form a complete portfolio by investing $8,000 in S&P 500 and $2,000 in the risk- free security. Given the information about S&P 500 and the risk-free security on the table, figure out an expected return, a standard deviation, and a beta for the complete portfolio. (40points) 5) A complete portfolio of $1,000 is composed of the risk-free security and a risky portfolio, P, constructed with two risky securities, X and Y. The optimal weights of X and Y in P are 80% and 20% respectively. Given the risk-free rate of 49. X has an expected return of 10%, and Y has an expected return of 12%. a) Figure out an expected return on the risky portfolio, P. (10points) b) To form a complete portfolio with an expected return of 72%, how much should you invest in the risk-free security, securities X and Y respectively? (30points) 1. Use the following information to answer the questions. Security Beta Standard Deviation Expected return S&P 500 20% 10.0% Risk-free security 0% 4.0% Stock D 30% 13.0% Stock E 15% ( )% Stock F 25% ( )% 1) Figure out the market risk premium using both S&P 500 and the risk-free security. (10points) 2) Figure out a beta for Stock D and an expected return for Stock E using the CAPM equation. (30points) 3) I Stock F has an average return of 12%, i) figure out the expected return based on the CAPM equation and the beta of 1.2. ii) figure out the abnormal return, alpha (a). (20points) 4) You form a complete portfolio by investing $8,000 in S&P 500 and $2,000 in the risk- free security. Given the information about S&P 500 and the risk-free security on the table, figure out an expected return, a standard deviation, and a beta for the complete portfolio. (40points) 5) A complete portfolio of $1,000 is composed of the risk-free security and a risky portfolio, P, constructed with two risky securities, X and Y. The optimal weights of X and Y in P are 80% and 20% respectively. Given the risk-free rate of 49. X has an expected return of 10%, and Y has an expected return of 12%. a) Figure out an expected return on the risky portfolio, P. (10points) b) To form a complete portfolio with an expected return of 72%, how much should you invest in the risk-free security, securities X and Y respectively? (30points)