Question
1. What probability rule applies to this scenario? If 58% of the stats studs surveyed have t-rex arms, what the the probability that a randomly
1. What probability rule applies to this scenario?
If 58% of the stats studs surveyed have "t-rex" arms, what the the probability that a randomly chosen stats stud would not have "t-rex" arms?
A.#3 the "add probabilities if there is no overlap" rule (aka "the disjoint addition rule")
B. #5 the "subtract off anything that overlaps" rule
C.#2 the "all together we are one" rule
D. #4 the complement rule (aka the most romantic rule because "together we make one")
E. #1 the "don't make an obvious mistake" rule
F.#6 the multiplication rule for independent events rule
2. Assuming that no one in the class is wearing wool socks with sandals, what is the probability of picking a random student who is either wearing wool socks or is wearing sandals?
A. #2 the "all together we are one" rule
B. #4 the complement rule (aka the most romantic rule because "together we make one")
C. #3 the "add probabilities if there is no overlap" rule (aka "the disjoint addition rule")
D. #5 the "subtract off anything that overlaps" rule
E. #1 the "don't make an obvious mistake" rule
F. #6 the multiplication rule for independent events rule
3. What probability rule applies to this scenario?
The probability of rolling a 5 on a standard 6-sided die is 1/6. The probability of rolling a 4 on the next roll is also 1/6. Each roll is independent from the last, therefore the probability of rolling a 5 and then a 4 is...
A. #3 the "add probabilities if there is no overlap" rule (aka "the disjoint addition rule")
B. #5 the "subtract off anything that overlaps" rule
C. #4 the complement rule (aka the most romantic rule because "together we make one")
D.#2 the "all together we are one" rule
#E. 6 the multiplication rule for independent events rule
F. #1 the "don't make an obvious mistake" rule
4. What probability rule applies to this scenario?
Consider these facts about the first assignment in a History 101 class.
The probability that a randomly chosen student got a passing grade on the assignment is 88%
The probability that a randomly chosen student got an A on the assignment is 10%
Question: What is the probability that a randomly chosen student got passing grade that wasn't an A?
A. #1 the "don't make an obvious mistake" rule
B. #6 the multiplication rule for independent events rule
C. #3 the "add probabilities if there is no overlap" rule (aka "the disjoint addition rule")
D. #2 the "all together we are one" rule
E. #4 the complement rule (aka the most romantic rule because "together we make one")
F. #5 the "subtract off anything that overlaps" rule
5. What probability rule applies to this scenario?
When Stephen goes grocery shopping they spend more than $20 for about 75% of the trips, and given into temptation to buy ice cream about 30% of the trips. Therefor the chance that they will only do one of those is:
P(getting ice cream)-P(spending more than $20 at grocery store)
= 30%-75%
=-45%
A. #2 the "all together we are one" rule
B. #5 the "subtract off anything that overlaps" rule
C. #6 the multiplication rule for independent events rule
D. #3 the "add probabilities if there is no overlap" rule (aka "the disjoint addition rule")
E. #1 the "don't make an obvious mistake" rule
F. #4 the complement rule (aka the most romantic rule because "together we make one")


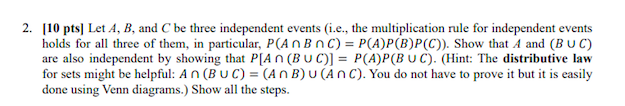
Step by Step Solution
There are 3 Steps involved in it
Step: 1

Get Instant Access to Expert-Tailored Solutions
See step-by-step solutions with expert insights and AI powered tools for academic success
Step: 2

Step: 3

Ace Your Homework with AI
Get the answers you need in no time with our AI-driven, step-by-step assistance
Get Started


