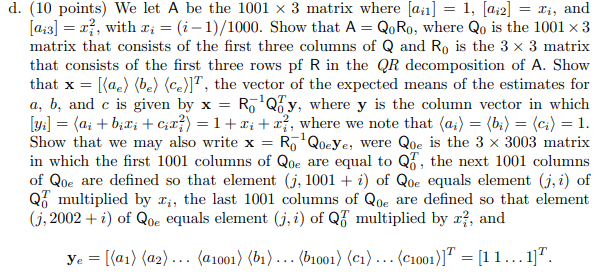
(10 points) We let A be the 1001 3 matrix where [ai1] = 1, [ai2] = xi , and [ai3] = x 2 i , with xi = (i1)/1000. Show that A = Q0R0, where Q0 is the 10013 matrix that consists of the first three columns of Q and R0 is the 3 3 matrix that consists of the first three rows pf R in the QR decomposition of A. Show that x = [ae be ce] T , the vector of the expected means of the estimates for a, b, and c is given by x = R 1 0 QT 0 y, where y is the column vector in which [yi ] = ai + bixi + cix 2 i = 1 + xi + x 2 i , where we note that ai = bi = ci = 1. Show that we may also write x = R 1 0 Q0eye, were Q0e is the 3 3003 matrix in which the first 1001 columns of Q0e are equal to QT 0 , the next 1001 columns of Q0e are defined so that element (j, 1001 + i) of Q0e equals element (j, i) of QT 0 multiplied by xi , the last 1001 columns of Q0e are defined so that element (j, 2002 + i) of Q0e equals element (j, i) of QT 0 multiplied by x 2 i , and ye = [a1 a2. . . a1001 b1. . .b1001 c1. . .c1001] T = [1 1 . . . 1]T .

\f